树与超积分洛特卡-伏特拉家族

IF 0.9 3区数学 Q3 MATHEMATICS, APPLIED

Mathematical Physics, Analysis and Geometry Pub Date : 2024-11-26 DOI:10.1007/s11040-024-09496-7

Peter H. van der Kamp, G. R. W. Quispel, David I. McLaren

{"title":"树与超积分洛特卡-伏特拉家族","authors":"Peter H. van der Kamp, G. R. W. Quispel, David I. McLaren","doi":"10.1007/s11040-024-09496-7","DOIUrl":null,"url":null,"abstract":"<div>To any tree on n vertices we associate an n-dimensional Lotka–Volterra system with \\(3n-2\\) parameters and, for generic values of the parameters, prove it is superintegrable, i.e. it admits \\(n-1\\) functionally independent integrals. We also show how each system can be reduced to an (\\(n-1\\))-dimensional system which is superintegrable and solvable by quadratures.</div>","PeriodicalId":694,"journal":{"name":"Mathematical Physics, Analysis and Geometry","volume":"27 4","pages":""},"PeriodicalIF":0.9000,"publicationDate":"2024-11-26","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Trees and Superintegrable Lotka–Volterra Families\",\"authors\":\"Peter H. van der Kamp, G. R. W. Quispel, David I. McLaren\",\"doi\":\"10.1007/s11040-024-09496-7\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<div>To any tree on n vertices we associate an n-dimensional Lotka–Volterra system with \\\\(3n-2\\\\) parameters and, for generic values of the parameters, prove it is superintegrable, i.e. it admits \\\\(n-1\\\\) functionally independent integrals. We also show how each system can be reduced to an (\\\\(n-1\\\\))-dimensional system which is superintegrable and solvable by quadratures.</div>\",\"PeriodicalId\":694,\"journal\":{\"name\":\"Mathematical Physics, Analysis and Geometry\",\"volume\":\"27 4\",\"pages\":\"\"},\"PeriodicalIF\":0.9000,\"publicationDate\":\"2024-11-26\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Mathematical Physics, Analysis and Geometry\",\"FirstCategoryId\":\"100\",\"ListUrlMain\":\"https://link.springer.com/article/10.1007/s11040-024-09496-7\",\"RegionNum\":3,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q3\",\"JCRName\":\"MATHEMATICS, APPLIED\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Mathematical Physics, Analysis and Geometry","FirstCategoryId":"100","ListUrlMain":"https://link.springer.com/article/10.1007/s11040-024-09496-7","RegionNum":3,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q3","JCRName":"MATHEMATICS, APPLIED","Score":null,"Total":0}

引用次数: 0

摘要

对于 n 个顶点上的任何树，我们都会关联一个具有 \(3n-2\) 个参数的 n 维 Lotka-Volterra 系统，并且对于参数的一般值，证明它是超可integrable 的，即它允许 \(n-1\) 个函数独立的积分。我们还展示了如何将每个系统还原为一个（\(n-1\)）维系统，该系统是超可解的，并且可以通过二次函数求解。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

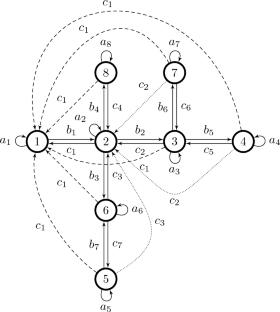
Trees and Superintegrable Lotka–Volterra Families

查看原文本刊更多论文

Trees and Superintegrable Lotka–Volterra Families

To any tree on n vertices we associate an n-dimensional Lotka–Volterra system with \(3n-2\) parameters and, for generic values of the parameters, prove it is superintegrable, i.e. it admits \(n-1\) functionally independent integrals. We also show how each system can be reduced to an (\(n-1\))-dimensional system which is superintegrable and solvable by quadratures.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Mathematical Physics, Analysis and Geometry 数学-物理：数学物理

CiteScore

2.10

自引率

0.00%

发文量

审稿时长

>12 weeks

期刊介绍： MPAG is a peer-reviewed journal organized in sections. Each section is editorially independent and provides a high forum for research articles in the respective areas. The entire editorial board commits itself to combine the requirements of an accurate and fast refereeing process. The section on Probability and Statistical Physics focuses on probabilistic models and spatial stochastic processes arising in statistical physics. Examples include: interacting particle systems, non-equilibrium statistical mechanics, integrable probability, random graphs and percolation, critical phenomena and conformal theories. Applications of probability theory and statistical physics to other areas of mathematics, such as analysis (stochastic pde''s), random geometry, combinatorial aspects are also addressed. The section on Quantum Theory publishes research papers on developments in geometry, probability and analysis that are relevant to quantum theory. Topics that are covered in this section include: classical and algebraic quantum field theories, deformation and geometric quantisation, index theory, Lie algebras and Hopf algebras, non-commutative geometry, spectral theory for quantum systems, disordered quantum systems (Anderson localization, quantum diffusion), many-body quantum physics with applications to condensed matter theory, partial differential equations emerging from quantum theory, quantum lattice systems, topological phases of matter, equilibrium and non-equilibrium quantum statistical mechanics, multiscale analysis, rigorous renormalisation group.