将高阶逻辑与集合论形式化相结合。

IF 0.9 3区计算机科学 Q4 COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCE

Journal of Automated Reasoning Pub Date : 2023-01-01 Epub Date: 2023-05-25 DOI:10.1007/s10817-023-09663-5

Cezary Kaliszyk, Karol Pąk

{"title":"将高阶逻辑与集合论形式化相结合。","authors":"Cezary Kaliszyk, Karol Pąk","doi":"10.1007/s10817-023-09663-5","DOIUrl":null,"url":null,"abstract":"The Isabelle Higher-order Tarski-Grothendieck object logic includes in its foundations both higher-order logic and set theory, which allows importing the libraries of Isabelle/HOL and Isabelle/Mizar. The two libraries, however, define all the basic concepts independently, which means that the results in the two are disconnected. In this paper, we align significant parts of these two libraries, by defining isomorphisms between their concepts, including the real numbers and algebraic structures. The isomorphisms allow us to transport theorems between the foundations and use the results from the libraries simultaneously.","PeriodicalId":15082,"journal":{"name":"Journal of Automated Reasoning","volume":"67 2","pages":"20"},"PeriodicalIF":0.9000,"publicationDate":"2023-01-01","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"https://www.ncbi.nlm.nih.gov/pmc/articles/PMC10209288/pdf/","citationCount":"0","resultStr":"{\"title\":\"Combining Higher-Order Logic with Set Theory Formalizations.\",\"authors\":\"Cezary Kaliszyk, Karol Pąk\",\"doi\":\"10.1007/s10817-023-09663-5\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"The Isabelle Higher-order Tarski-Grothendieck object logic includes in its foundations both higher-order logic and set theory, which allows importing the libraries of Isabelle/HOL and Isabelle/Mizar. The two libraries, however, define all the basic concepts independently, which means that the results in the two are disconnected. In this paper, we align significant parts of these two libraries, by defining isomorphisms between their concepts, including the real numbers and algebraic structures. The isomorphisms allow us to transport theorems between the foundations and use the results from the libraries simultaneously.\",\"PeriodicalId\":15082,\"journal\":{\"name\":\"Journal of Automated Reasoning\",\"volume\":\"67 2\",\"pages\":\"20\"},\"PeriodicalIF\":0.9000,\"publicationDate\":\"2023-01-01\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"https://www.ncbi.nlm.nih.gov/pmc/articles/PMC10209288/pdf/\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Journal of Automated Reasoning\",\"FirstCategoryId\":\"94\",\"ListUrlMain\":\"https://doi.org/10.1007/s10817-023-09663-5\",\"RegionNum\":3,\"RegionCategory\":\"计算机科学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"2023/5/25 0:00:00\",\"PubModel\":\"Epub\",\"JCR\":\"Q4\",\"JCRName\":\"COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCE\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Journal of Automated Reasoning","FirstCategoryId":"94","ListUrlMain":"https://doi.org/10.1007/s10817-023-09663-5","RegionNum":3,"RegionCategory":"计算机科学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"2023/5/25 0:00:00","PubModel":"Epub","JCR":"Q4","JCRName":"COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCE","Score":null,"Total":0}

引用次数: 0

摘要

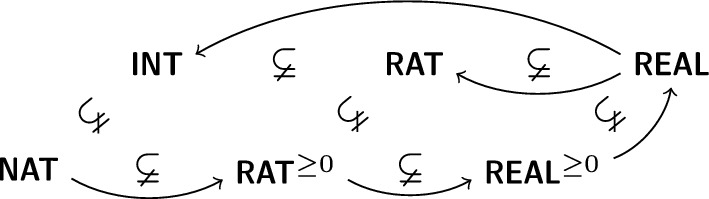
Isabelle高阶Tarski Grothendieck对象逻辑在其基础中包括高阶逻辑和集合论，这允许导入Isabelle/HOL和Isabelle/Mizar的库。然而，这两个库独立地定义了所有的基本概念，这意味着两者中的结果是不相连的。在本文中，我们通过定义它们的概念之间的同构，包括实数和代数结构，来对齐这两个库的重要部分。同构允许我们在基础之间传递定理，并同时使用库的结果。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

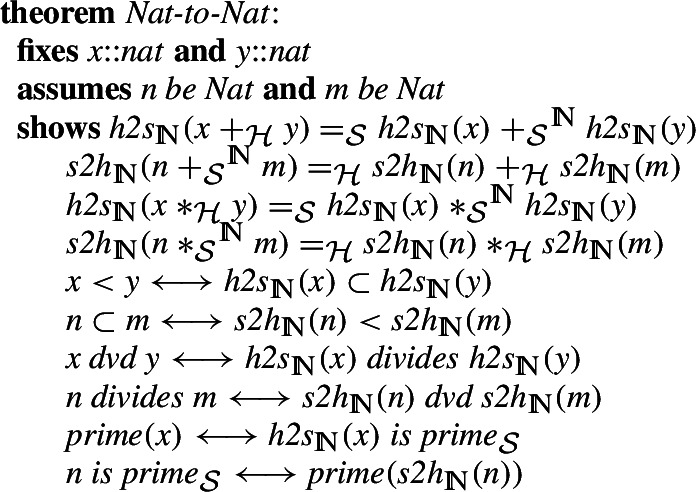
Combining Higher-Order Logic with Set Theory Formalizations.

查看原文本刊更多论文

Combining Higher-Order Logic with Set Theory Formalizations.

The Isabelle Higher-order Tarski-Grothendieck object logic includes in its foundations both higher-order logic and set theory, which allows importing the libraries of Isabelle/HOL and Isabelle/Mizar. The two libraries, however, define all the basic concepts independently, which means that the results in the two are disconnected. In this paper, we align significant parts of these two libraries, by defining isomorphisms between their concepts, including the real numbers and algebraic structures. The isomorphisms allow us to transport theorems between the foundations and use the results from the libraries simultaneously.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Journal of Automated Reasoning 工程技术-计算机：人工智能

CiteScore

3.60

自引率

9.10%

发文量

审稿时长

>12 weeks

期刊介绍： The Journal of Automated Reasoning is an interdisciplinary journal that maintains a balance between theory, implementation and application. The spectrum of material published ranges from the presentation of a new inference rule with proof of its logical properties to a detailed account of a computer program designed to solve various problems in industry. The main fields covered are automated theorem proving, logic programming, expert systems, program synthesis and validation, artificial intelligence, computational logic, robotics, and various industrial applications. The papers share the common feature of focusing on several aspects of automated reasoning, a field whose objective is the design and implementation of a computer program that serves as an assistant in solving problems and in answering questions that require reasoning. The Journal of Automated Reasoning provides a forum and a means for exchanging information for those interested purely in theory, those interested primarily in implementation, and those interested in specific research and industrial applications.