参数各向异性（p，q）-Neumann问题

IF 0.9 4区数学 Q3 MATHEMATICS, APPLIED

Acta Mathematicae Applicatae Sinica, English Series Pub Date : 2023-11-08 DOI:10.1007/s10255-023-1087-y

Zhen-hai Liu, Nikolaos S. Papageorgiou

{"title":"参数各向异性（p，q）-Neumann问题","authors":"Zhen-hai Liu, Nikolaos S. Papageorgiou","doi":"10.1007/s10255-023-1087-y","DOIUrl":null,"url":null,"abstract":"<div><p>We consider a Neumann problem driven by a (<i>p</i>(<i>z</i>), <i>q</i>(<i>z</i>))-Laplacian (anisotropic problem) plus a parametric potential term with λ > 0 being the parameter. The reaction is superlinear but need not satisfy the Ambrosetti-Rabinowitz condition. We prove a bifurcation-type theorem describing the changes in the set of positive solutions as the parameter λ moves on </p><div><figure><div><div><picture><source><img></source></picture></div></div></figure></div><p>.</p></div>","PeriodicalId":6951,"journal":{"name":"Acta Mathematicae Applicatae Sinica, English Series","volume":"39 4","pages":"926 - 942"},"PeriodicalIF":0.9000,"publicationDate":"2023-11-08","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Parametric Anisotropic (p, q)-Neumann Problems\",\"authors\":\"Zhen-hai Liu, Nikolaos S. Papageorgiou\",\"doi\":\"10.1007/s10255-023-1087-y\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<div><p>We consider a Neumann problem driven by a (<i>p</i>(<i>z</i>), <i>q</i>(<i>z</i>))-Laplacian (anisotropic problem) plus a parametric potential term with λ > 0 being the parameter. The reaction is superlinear but need not satisfy the Ambrosetti-Rabinowitz condition. We prove a bifurcation-type theorem describing the changes in the set of positive solutions as the parameter λ moves on </p><div><figure><div><div><picture><source><img></source></picture></div></div></figure></div><p>.</p></div>\",\"PeriodicalId\":6951,\"journal\":{\"name\":\"Acta Mathematicae Applicatae Sinica, English Series\",\"volume\":\"39 4\",\"pages\":\"926 - 942\"},\"PeriodicalIF\":0.9000,\"publicationDate\":\"2023-11-08\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Acta Mathematicae Applicatae Sinica, English Series\",\"FirstCategoryId\":\"100\",\"ListUrlMain\":\"https://link.springer.com/article/10.1007/s10255-023-1087-y\",\"RegionNum\":4,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q3\",\"JCRName\":\"MATHEMATICS, APPLIED\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Acta Mathematicae Applicatae Sinica, English Series","FirstCategoryId":"100","ListUrlMain":"https://link.springer.com/article/10.1007/s10255-023-1087-y","RegionNum":4,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q3","JCRName":"MATHEMATICS, APPLIED","Score":null,"Total":0}

引用次数: 0

摘要

我们考虑由（p（z），q（z））-拉普拉斯（各向异性问题）加上λ>；0是参数。反应是超线性的，但不需要满足Ambrosetti-Rabinowitz条件。我们证明了一个分叉型定理，该定理描述了正解集随着参数λ的移动而发生的变化。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

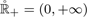
Parametric Anisotropic (p, q)-Neumann Problems

查看原文本刊更多论文

Parametric Anisotropic (p, q)-Neumann Problems

We consider a Neumann problem driven by a (p(z), q(z))-Laplacian (anisotropic problem) plus a parametric potential term with λ > 0 being the parameter. The reaction is superlinear but need not satisfy the Ambrosetti-Rabinowitz condition. We prove a bifurcation-type theorem describing the changes in the set of positive solutions as the parameter λ moves on

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Acta Mathematicae Applicatae Sinica, English Series 数学-应用数学

CiteScore

1.30

自引率

0.00%

发文量

审稿时长

3.0 months

期刊介绍： Acta Mathematicae Applicatae Sinica (English Series) is a quarterly journal established by the Chinese Mathematical Society. The journal publishes high quality research papers from all branches of applied mathematics, and particularly welcomes those from partial differential equations, computational mathematics, applied probability, mathematical finance, statistics, dynamical systems, optimization and management science.