基于奇异核的新型冠状病毒模型分数阶数学模型的理论与数值分析
IF 0.8
4区 综合性期刊
Q3 MULTIDISCIPLINARY SCIENCES
Proceedings of the National Academy of Sciences, India Section A: Physical Sciences
Pub Date : 2023-01-09
DOI:10.1007/s40010-022-00805-9
引用次数: 2
摘要
本研究提出了冠状病毒的分数阶数学模型。我们选择COVID-19模型并将模型转换为分数阶。讨论了其理论和数值分析。首先,利用不动点定理研究了分数阶COVID-19模型的存在唯一性结果。此外,我们还利用Hyers-Ulam稳定性进行了稳定性分析。分数运算符用于卡普托意义。我们使用著名的数值方法得到数值解,并使用所采用的数值方法给出图形解释。最后,我们对上述方法进行了比较,并根据得到的解进行了观察。本文章由计算机程序翻译,如有差异,请以英文原文为准。
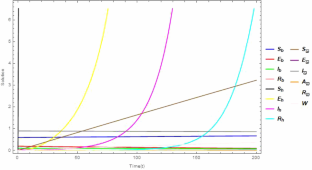
Theoretical and Numerical Analysis of Fractional Order Mathematical Model on Recent COVID-19 Model Using Singular Kernel
This study presents a fractional-order mathematical model of coronavirus. We select COVID-19 model and convert the model into fractional order. Discuss its theoretical and numerical analysis. Firstly, we investigate the existence and uniqueness results using some fixed point theorems for the proposed fractional-order COVID-19 model. Further, we provide the stability analysis with the help of the Hyers-Ulam stability. The fractional operator is used in the Caputo sense. We obtain numerical solutions using famous numerical methods and provide a graphical interpretation using adopted numerical methods. Finally, we compare the above techniques and provide observations according to the obtained solutions.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
2.60
自引率
0.00%
发文量
37
审稿时长
>12 weeks
期刊介绍:
To promote research in all the branches of Science & Technology; and disseminate the knowledge and advancements in Science & Technology

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


