直线粒子平面运动的逃逸盆地(3 +\documentclass[12pt]{minimal} \ uspackage {amsmath} \ uspackage {wasysym} \ uspackage {amsfonts} \ uspackage {amssymb} \ uspackage {amssysy} \ uspackage {mathrsfs} \ uspackage {upgreek} \setlength{\oddsidemargin}{-69pt} \begin{document}

IF 1.7 4区物理与天体物理 Q2 PHYSICS, MULTIDISCIPLINARY

Few-Body Systems Pub Date : 2023-07-31 DOI:10.1007/s00601-023-01852-7

Ibrahim Belgharbi, Juan F. Navarro

{"title":"直线粒子平面运动的逃逸盆地(3 +\\documentclass[12pt]{minimal} \\ uspackage {amsmath} \\ uspackage {wasysym} \\ uspackage {amsfonts} \\ uspackage {amssymb} \\ uspackage {amssysy} \\ uspackage {mathrsfs} \\ uspackage {upgreek} \\setlength{\\oddsidemargin}{-69pt} \\begin{document}","authors":"Ibrahim Belgharbi, Juan F. Navarro","doi":"10.1007/s00601-023-01852-7","DOIUrl":null,"url":null,"abstract":"<div><p>The objective of this work is to study the motion of an infinitesimal particle in the gravitational field of three big bodies in a ring configuration consisting of two peripheral and one central bodies, when the energy of the particle does not allow the escape from the potential well of the system. We have numerically determined the basins of escape using a new surface of section. Additionally, we have computed and analyzed the geometry of the set of asymptotic trajectories of the periodic orbit that governs the escape from the neighborhood of one of the two satellites, which also defines the limiting curves of the basins of escape from this region.\n</p></div>","PeriodicalId":556,"journal":{"name":"Few-Body Systems","volume":"64 3","pages":""},"PeriodicalIF":1.7000,"publicationDate":"2023-07-31","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"https://link.springer.com/content/pdf/10.1007/s00601-023-01852-7.pdf","citationCount":"0","resultStr":"{\"title\":\"Basins of Escape of the Particle’s Planar Motion in the Rectilinear (3 \\\$\\\\varvec{+}\\\$ 1)-Body Ring Problem\",\"authors\":\"Ibrahim Belgharbi, Juan F. Navarro\",\"doi\":\"10.1007/s00601-023-01852-7\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<div><p>The objective of this work is to study the motion of an infinitesimal particle in the gravitational field of three big bodies in a ring configuration consisting of two peripheral and one central bodies, when the energy of the particle does not allow the escape from the potential well of the system. We have numerically determined the basins of escape using a new surface of section. Additionally, we have computed and analyzed the geometry of the set of asymptotic trajectories of the periodic orbit that governs the escape from the neighborhood of one of the two satellites, which also defines the limiting curves of the basins of escape from this region.\\n</p></div>\",\"PeriodicalId\":556,\"journal\":{\"name\":\"Few-Body Systems\",\"volume\":\"64 3\",\"pages\":\"\"},\"PeriodicalIF\":1.7000,\"publicationDate\":\"2023-07-31\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"https://link.springer.com/content/pdf/10.1007/s00601-023-01852-7.pdf\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Few-Body Systems\",\"FirstCategoryId\":\"101\",\"ListUrlMain\":\"https://link.springer.com/article/10.1007/s00601-023-01852-7\",\"RegionNum\":4,\"RegionCategory\":\"物理与天体物理\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q2\",\"JCRName\":\"PHYSICS, MULTIDISCIPLINARY\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Few-Body Systems","FirstCategoryId":"101","ListUrlMain":"https://link.springer.com/article/10.1007/s00601-023-01852-7","RegionNum":4,"RegionCategory":"物理与天体物理","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q2","JCRName":"PHYSICS, MULTIDISCIPLINARY","Score":null,"Total":0}

引用次数: 0

摘要

本文的目的是研究一个无限小粒子在由两个外围体和一个中心体组成的环形三大体的引力场中，当粒子的能量不允许从系统的势阱中逃逸时的运动。我们用一种新的剖面面数值确定了逃逸盆地。此外，我们还计算并分析了周期轨道的渐近轨迹集的几何形状，这些轨迹集控制着两颗卫星中的一颗从邻近区域的逃逸，它还定义了该区域逃逸盆地的极限曲线。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

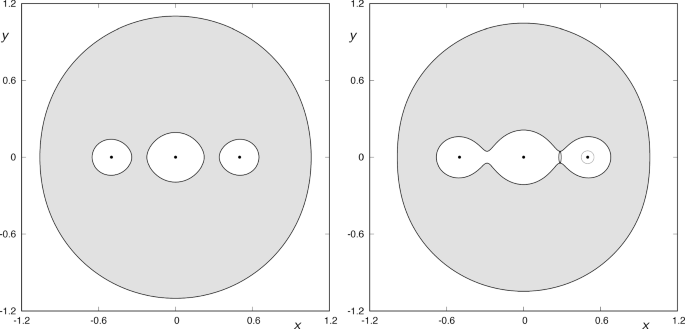
$Basins of Escape of the Particle’s Planar Motion in the Rectilinear (3 $\varvec{+}$ 1)-Body Ring Problem$

查看原文本刊更多论文

Basins of Escape of the Particle’s Planar Motion in the Rectilinear (3 $\varvec{+}$ 1)-Body Ring Problem

The objective of this work is to study the motion of an infinitesimal particle in the gravitational field of three big bodies in a ring configuration consisting of two peripheral and one central bodies, when the energy of the particle does not allow the escape from the potential well of the system. We have numerically determined the basins of escape using a new surface of section. Additionally, we have computed and analyzed the geometry of the set of asymptotic trajectories of the periodic orbit that governs the escape from the neighborhood of one of the two satellites, which also defines the limiting curves of the basins of escape from this region.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Few-Body Systems 物理-物理：综合

CiteScore

2.90

自引率

18.80%

发文量

审稿时长

6-12 weeks

期刊介绍： The journal Few-Body Systems presents original research work – experimental, theoretical and computational – investigating the behavior of any classical or quantum system consisting of a small number of well-defined constituent structures. The focus is on the research methods, properties, and results characteristic of few-body systems. Examples of few-body systems range from few-quark states, light nuclear and hadronic systems; few-electron atomic systems and small molecules; and specific systems in condensed matter and surface physics (such as quantum dots and highly correlated trapped systems), up to and including large-scale celestial structures. Systems for which an equivalent one-body description is available or can be designed, and large systems for which specific many-body methods are needed are outside the scope of the journal. The journal is devoted to the publication of all aspects of few-body systems research and applications. While concentrating on few-body systems well-suited to rigorous solutions, the journal also encourages interdisciplinary contributions that foster common approaches and insights, introduce and benchmark the use of novel tools (e.g. machine learning) and develop relevant applications (e.g. few-body aspects in quantum technologies).