神经场自组织映射的稳定性分析。
IF 2.3
4区 医学
Q1 Neuroscience
引用次数: 2
摘要
我们提供了保证自组织映射有效地开发输入空间表示的理论条件。这项研究依赖于初级体感皮层3b区时空活动的神经场模型。我们依靠李亚普诺夫的神经场理论来推导稳定性的理论条件。通过数值实验验证了理论条件。分析强调了横向突触耦合的兴奋和抑制之间的平衡以及突触增益的强度在自组织图谱的形成和维持中发挥的关键作用。本文章由计算机程序翻译,如有差异,请以英文原文为准。
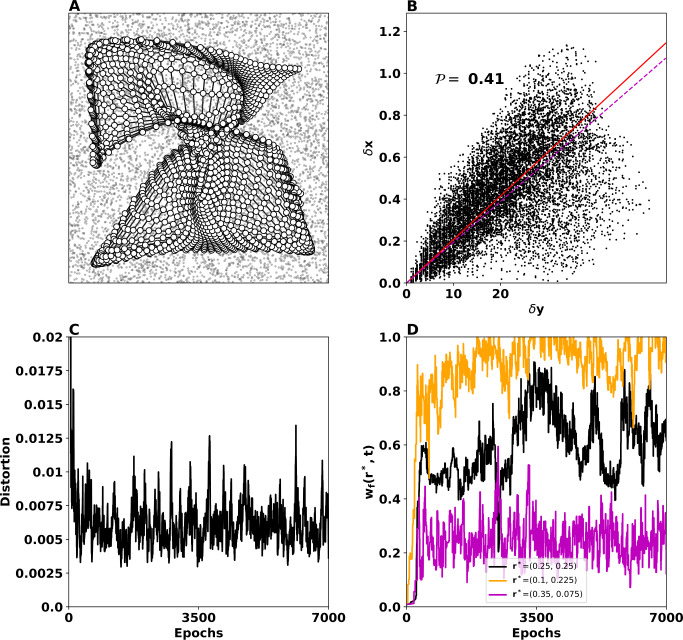
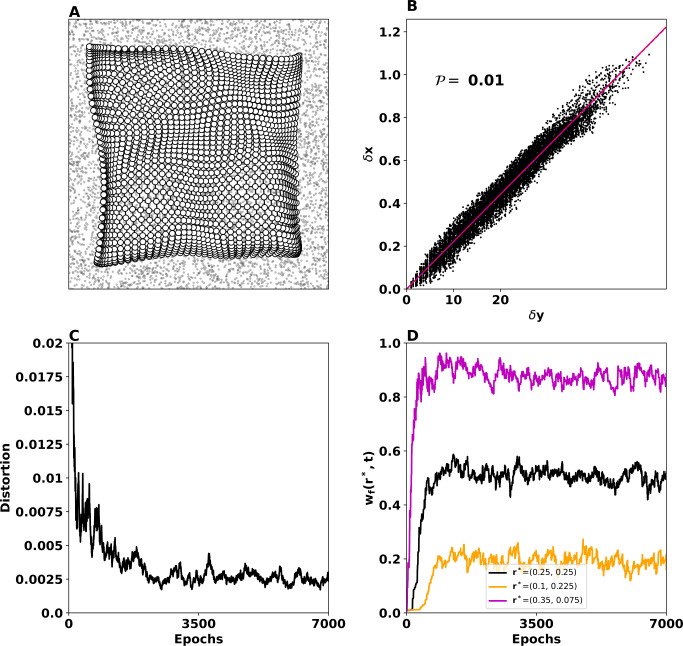
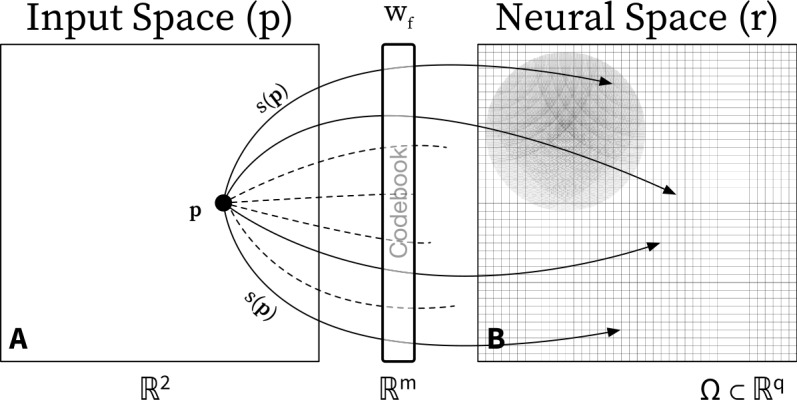
Stability analysis of a neural field self-organizing map.
We provide theoretical conditions guaranteeing that a self-organizing map efficiently develops representations of the input space. The study relies on a neural field model of spatiotemporal activity in area 3b of the primary somatosensory cortex. We rely on Lyapunov's theory for neural fields to derive theoretical conditions for stability. We verify the theoretical conditions by numerical experiments. The analysis highlights the key role played by the balance between excitation and inhibition of lateral synaptic coupling and the strength of synaptic gains in the formation and maintenance of self-organizing maps.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Journal of Mathematical Neuroscience
Neuroscience-Neuroscience (miscellaneous)
自引率
0.00%
发文量
0
审稿时长
13 weeks
期刊介绍:
The Journal of Mathematical Neuroscience (JMN) publishes research articles on the mathematical modeling and analysis of all areas of neuroscience, i.e., the study of the nervous system and its dysfunctions. The focus is on using mathematics as the primary tool for elucidating the fundamental mechanisms responsible for experimentally observed behaviours in neuroscience at all relevant scales, from the molecular world to that of cognition. The aim is to publish work that uses advanced mathematical techniques to illuminate these questions.
It publishes full length original papers, rapid communications and review articles. Papers that combine theoretical results supported by convincing numerical experiments are especially encouraged.
Papers that introduce and help develop those new pieces of mathematical theory which are likely to be relevant to future studies of the nervous system in general and the human brain in particular are also welcome.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


