消除行人密度估算中的偏差:从 Voronoi 单元的角度看问题
IF 2.8
3区 物理与天体物理
Q2 PHYSICS, MULTIDISCIPLINARY
Physica A: Statistical Mechanics and its Applications
Pub Date : 2024-11-23
DOI:10.1016/j.physa.2024.130251
引用次数: 0
摘要
对于不受空间限制的行人,人们一直致力于开发密度估算方法。在本文中,我们提出了一种基于 Voronoi 单元的新方法,为估算小型无边界行人群体中个体的密度提供了一种手段。对现有方法(包括在类似情况下使用的拉格朗日方法和欧拉方法)的全面评估发现了明显的局限性。具体来说,这些方法对于沿行人轨迹进行现实密度估算的定义不清,表现出系统性偏差和波动,而这些偏差和波动又取决于参数的选择。因此,需要一种与参数无关的方法来消除这种偏差。我们建议对广泛使用的基于 Voronoi 单元的密度估算进行修改,以适应不同规模的行人群体。这种改进的 Voronoi 方法的优点在于,它是一种瞬时方法,只需知道行人在给定时间的位置,不依赖于参数值的选择,能为我们提供个人附近密度的实际估计值,并且对各种情况下的小型和大型人群都具有适当的物理意义。最后,我们就密度测量对小型行人群体的意义发表一些一般性意见。本文章由计算机程序翻译,如有差异,请以英文原文为准。
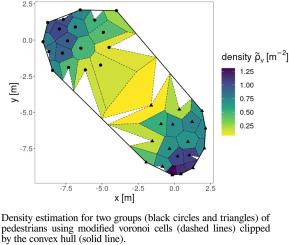
Eliminating bias in pedestrian density estimation: A Voronoi cell perspective
For pedestrians moving without spatial constraints, extensive research has been devoted to develop methods of density estimation. In this paper we present a new approach based on Voronoi cells, offering a means to estimate density for individuals in small, unbounded pedestrian groups. A thorough evaluation of existing methods, encompassing both Lagrangian and Eulerian approaches employed in similar contexts, reveals notable limitations. Specifically, these methods turn out to be ill-defined for realistic density estimation along a pedestrian’s trajectory, exhibiting systematic biases and fluctuations that depend on the choice of parameters. There is thus a need for a parameter-independent method to eliminate this bias. We propose a modification of the widely used Voronoi-cell based density estimate to accommodate pedestrian groups, irrespective of their size. The advantages of this modified Voronoi method are that it is an instantaneous method that requires only knowledge of the pedestrians’ positions at a give time, does not depend on the choice of parameter values, gives us a realistic estimate of density in an individual’s neighborhood, and has appropriate physical meaning for both small and large human crowds in a wide variety of situations. We conclude with general remarks about the meaning of density measurements for small groups of pedestrians.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
7.20
自引率
9.10%
发文量
852
审稿时长
6.6 months
期刊介绍:
Physica A: Statistical Mechanics and its Applications
Recognized by the European Physical Society
Physica A publishes research in the field of statistical mechanics and its applications.
Statistical mechanics sets out to explain the behaviour of macroscopic systems by studying the statistical properties of their microscopic constituents.
Applications of the techniques of statistical mechanics are widespread, and include: applications to physical systems such as solids, liquids and gases; applications to chemical and biological systems (colloids, interfaces, complex fluids, polymers and biopolymers, cell physics); and other interdisciplinary applications to for instance biological, economical and sociological systems.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


