百日咳 SVEIQRP 模型的分形分数微分方程系统动力学行为
Q1 Mathematics
Partial Differential Equations in Applied Mathematics
Pub Date : 2024-11-19
DOI:10.1016/j.padiff.2024.100990
引用次数: 0
摘要
这项工作利用了分形分微分方程系统的应用。非整数阶的传染病模型吸引了众多数学家和生物科学家。有必要提出一种先进的分形分数模型,用于研究流行病学中传染病的精确动力学行为。本研究提出了百日咳数学模型的新版本。百日咳是一种疾病,可通过接触、咳嗽和空气飞沫等多种途径在人与人之间传播。在这项研究中,我们考虑了百日咳模型,并增加了一个新的永久隔室。加入永久恢复区后,我们修改后的模型能更好地解释对百日咳产生长期免疫力的个体。对模型的这一补充增强了模型在现实世界中的准确性。我们使用分形分数微分方程系统分析了所考虑的模型,并对研究结果进行了数值模拟。利用定点定理确定了模型的存在性、唯一性和 Hyers-Ulam 稳定性。模型动力学行为的数值结果也记录在表格中。本文章由计算机程序翻译,如有差异,请以英文原文为准。
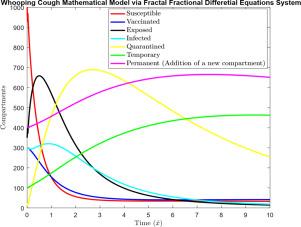
Dynamical behavior of whooping cough SVEIQRP model via system of fractal fractional differential equations
In this work, the application of the system of fractal fractional differential equations is exploited. Infectious diseases modeling of non-integer order attracts many mathematicians and biological scientists. The presentation of an advanced fractal fractional model for studying the exact dynamical behavior of infectious diseases in epidemiology is necessary. In this study, a new version of a mathematical model for whooping cough is presented. Whooping cough is a disease and can be transmitted from humans to humans through various means, i.e., touch, cough, and air droplets. In this study, we considered the whooping cough model with a new permanent compartment. Our modified model after incorporating the permanent recovered compartment explains better regarding the individuals who have developed long term immunity against whooping cough. This addition to the model enhances the model’s accuracy towards real world scenarios. The considered model was analyzed by using a system of fractal fractional differential equations, and numerical simulation was established for the findings of this study. The fixed point theorem was used to determine the existence, uniqueness, and Hyers–Ulam stability of the model. Numerical results of the dynamical behavior of the model are also recorded in tables.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Partial Differential Equations in Applied Mathematics
Mathematics-Analysis
CiteScore
6.20
自引率
0.00%
发文量
138
审稿时长
14 weeks
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


