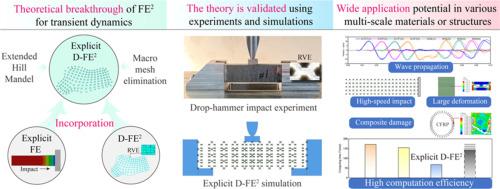
An explicit D-FE2 method for transient multiscale analysis

Explicit FE (Finite Element) method offers distinct advantages for a variety of simulations, including nonlinear transient dynamics, large deformation due to buckling, and damage evolution in materials or structures. However, conventional computational homogenization techniques, such as the FE² and direct FE² (D-FE²) methods, have not yet been integrated with an explicit algorithm because of the implicit framework in their numerical implementation, and thus cannot be widely applied to concurrent multi-level modeling of transient dynamic issues in multiscale materials and structures. In this study, an explicit D-FE² method was proposed by incorporating explicit integration algorithms into the numerical calculation of microscale RVEs based on the D-FE² method proposed by Tan [1]. To facilitate this, an extended Hill–Mandel principle which considers the conservation of both kinetic and internal energies between macro- and micro-scales was derived, and the conventional D-FE² method was modified using the explicit FE method. The proposed explicit D-FE² method was validated using a series of experiments and numerical examples including drop-hammer impact on multiscale honeycomb, stress wave propagation in porous materials, compressive buckling of multi-stable metamaterials, damage and failure of fiber-reinforced composites, etc. It was validated that the proposed explicit D-FE² method is feasible and efficient for transient dynamic analysis of multiscale materials and structures, which might be a new avenue of research in the field of impact dynamics.