阐明氢分子 nH2(n = 1 ÷ 7)基态电子项莫尔斯近似中的同位素效应。展示赫茨伯格反常现象和非谐波性。
IF 4.6
2区 化学
Q1 SPECTROSCOPY
Spectrochimica Acta Part A: Molecular and Biomolecular Spectroscopy
Pub Date : 2024-11-08
DOI:10.1016/j.saa.2024.125410
引用次数: 0
摘要
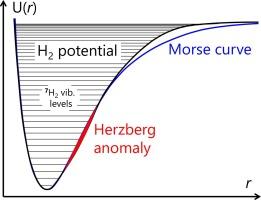
莫尔斯函数是二原子分子势的最简单的非谐波近似值,几乎可以精确地求解振动薛定谔方程。尽管莫尔斯函数很粗糙,但它已被广泛用于解决分子光谱学中的各种问题。近年来,人们特别关注二原子分子电子项 U(r)的两种莫尔斯近似值 M1(r) 和 M2(r),它们通过选择主要拟合参数而有所不同,并能令人满意地再现 U(r)的不同部分。通过考虑差分函数 U(r) - M(r),可以突出简单项 U(r)的一些细微特征,其形式与莫尔斯函数差别不大。莫尔斯近似预测的振动级集合 G(υ) 可以用差分函数 Δ1G(υ) 和 Δ2G(υ) 方便地表征,它们分别是振动量子的能量及其变化率。函数 Δ2G(υ)可以看作是对非谐调常数的概括,而非谐调常数是由实验中的水平位置 υ = 0,1,2 决定的。最后,在分析非谐波性时,比较不同同位素的结果是有用的,对于这些同位素,U(r) 是相同的,但振动级的密度是不同的。氢有七种已知同位素,其中包括寿命极短的同位素,因此氢的同位素效应范围最大。在这项工作中,针对氢分子 nH2 的七种同位素(n = 1-7),研究了还原质量对莫尔斯函数近似基态电子势 U(r) 结果的影响。对于每种同位素,都考虑了 M1(r) 和 M2(r) 两种近似值。M1(r) 与 U(r) 的系统偏差随 r 的增加而增大,在渐近线区域达到 1700-2770 cm-1(对于 7H2-1H2)。M2(r) 与 U(r) 的系统偏差在距离越大时越小:它呈圆顶形,在 r ∼ 2 Å 时达到约 1500 cm-1 的最大值,之后下降为零。不同同位素的 M2(r) 曲线相差不到 20 cm-1。U(r) - M(r) 函数用于分析 M1(r) 和 M2(r) 与 U(r) 相交区域的所谓 "赫茨伯格异常"。例如,在 r = 1.08 Å 附近,U(r) - M2(r) 函数显示了从 -125 cm-1 (1H2)到 -142 cm-1 (7H2)的异常负值。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Elucidating the isotope effect in the Morse approximation of the ground state electronic term of hydrogen molecules nH2, n = 1 ÷ 7. Showcasing the Herzberg anomaly and anharmonicity
The Morse function is the simplest anharmonic approximation of the potential of a diatomic molecule, for which the vibrational Schrödinger equation could be solved almost precisely. Despite its crudeness, the Morse function has been widely used in solving various problems in molecular spectroscopy. In recent years, special attention was paid to the existence of two Morse approximations for the electronic terms U(r) of diatomic molecules, M1(r) and M2(r), which differ by the selection of primary fitting parameters and satisfactorily reproduce different parts of U(r). Some fine features of simple terms U(r), whose form does not differ much from the Morse function, could be highlighted by considering difference functions U(r) − M(r). The set of vibrational levels G(υ), predicted by Morse approximation, can be conveniently characterized by the difference functions Δ1G(υ) and Δ2G(υ), which are, respectively, the energies of vibrational quanta and the rates of their change. The function Δ2G(υ) can be considered as a generalization of the anharmonicity constant, determined experimentally by the position of levels υ = 0,1,2. Finally, analysing the anharmonicity, it is useful to compare the results obtained for various isotopologues, for which U(r) is the same, but the density of vibrational levels is different. The maximal range of isotope effects is expected for hydrogen, which has seven known isotopes, including extremely short-lived ones. In this work, the influence of the reduced mass on the results of the approximation of the ground state electronic potential U(r) by the Morse function is studied for seven isotopologues of hydrogen molecule nH2 (n = 1–7). For each isotopologue, alternative approximations M1(r) and M2(r) are considered. The systematic deviation of M1(r) from U(r) increases with r, reaching 1700–2770 cm−1 (for 7H2–1H2) in the region of the asymptote. The systematic deviation of M2(r) from U(r) decreases at larger distances: it is dome-shaped with a maximum of ca. 1500 cm−1 at r ∼ 2 Å, after which it falls to zero. The M2(r) curves for different isotopologues diverge by less than 20 cm−1. The U(r) − M(r) functions are used to analyse the so-called “Herzberg anomaly” in the region where M1(r) and M2(r) intersect U(r). For example, at around r = 1.08 Å the U(r) − M2(r) function shows anomalous negative values ranging from −125 cm−1 (for 1H2) to −142 cm−1 (for 7H2).
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
8.40
自引率
11.40%
发文量
1364
审稿时长
40 days
期刊介绍:
Spectrochimica Acta, Part A: Molecular and Biomolecular Spectroscopy (SAA) is an interdisciplinary journal which spans from basic to applied aspects of optical spectroscopy in chemistry, medicine, biology, and materials science.
The journal publishes original scientific papers that feature high-quality spectroscopic data and analysis. From the broad range of optical spectroscopies, the emphasis is on electronic, vibrational or rotational spectra of molecules, rather than on spectroscopy based on magnetic moments.
Criteria for publication in SAA are novelty, uniqueness, and outstanding quality. Routine applications of spectroscopic techniques and computational methods are not appropriate.
Topics of particular interest of Spectrochimica Acta Part A include, but are not limited to:
Spectroscopy and dynamics of bioanalytical, biomedical, environmental, and atmospheric sciences,
Novel experimental techniques or instrumentation for molecular spectroscopy,
Novel theoretical and computational methods,
Novel applications in photochemistry and photobiology,
Novel interpretational approaches as well as advances in data analysis based on electronic or vibrational spectroscopy.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


