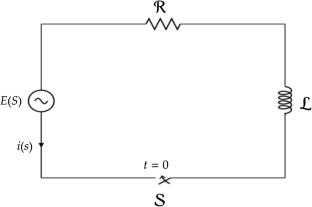
Mohand Transform Approach to Ulam-Type Stability of Linear Differential Equations

This study applies the Mohand transform approach to analyze stability results, specifically Ulam-Hyers stability of linear differential equations. We thoroughly examine the theoretical results and support them with well-fitted examples. We also establish the electrical circuit using the proposed method from an application standpoint.