具有流体动力学相互作用和颗粒惯性的沉积悬浮液中的分散和颗粒压力
IF 3.6
2区 工程技术
Q1 MECHANICS
International Journal of Multiphase Flow
Pub Date : 2024-10-25
DOI:10.1016/j.ijmultiphaseflow.2024.105037
引用次数: 0
摘要
粒子惯性是悬浮动力学中经常被忽视的一个特征。然而,对它的忽视会改变粒子运动控制方程的阶次。在这项工作中,我们研究了颗粒惯性对其速度波动和由此产生的应力的影响。为了观察粒子惯性的影响,我们考虑了在低雷诺数下牛顿流体中沉积的球形弱布朗微粒子的尘气悬浮液。我们首先研究单个球形粒子的运动。通过对这一简单情况的模拟,我们可以定义适当的流动物理参数,并验证在佩克莱特数和斯托克斯数范围内的速度统计数值计算。接下来,我们利用周期性边界条件进行朗之万动力学模拟,对悬浮在粘性流体中的 N 个流体力学相互作用粒子的平移和旋转运动方程进行积分。本文的第一个目的是研究惯性粒子的速度方差行为,在中等佩克莱特数下布朗运动产生的小波动。轻度布朗力和流体动力学相互作用降低了在沉积颗粒的非布朗悬浮液(Pe≫1)中观察到的速度波动的各向异性。模拟结果表明,颗粒的惯性减弱了布朗运动和粘性流体力学相互作用产生的速度波动幅度。此外,我们还通过计算速度波动的自相关函数和颗粒扩散率,研究了速度波动的长期行为。数值模拟结果表明,悬浮液中的流体动力相互作用产生的流动扰动会产生明显的颗粒压力。根据本数值模拟中获得的粒子速度方差,我们提出了一个简单的粒子相压力模型,即粒子体积分数 ϕ⩽5%的线性函数,这通常是流化床等更复杂粒子系统模型所需的闭合量。本文章由计算机程序翻译,如有差异,请以英文原文为准。
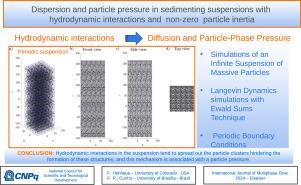
Dispersion and particle pressure in sedimenting suspensions with hydrodynamic interactions and particle inertia
Particle inertia is a feature of suspension dynamics that is often neglected. However, its neglect changes the order of the governing equations of particle motion. In this work, we examine the impact of the inertia of particles over their velocity fluctuations and the resulting stresses. In order to observe effects of particle inertia, we consider dusty-gas suspensions of spherical weakly Brownian microparticles sedimenting in a Newtonian fluid at low Reynolds numbers. We first investigate the motion of a single spherical particle. The simulations for this simple case allow us to define the appropriate physical parameters of the flow and to validate the numerical calculation of velocity statistics over a range of Péclet and Stokes numbers. Next, we perform Langevin dynamics simulations with periodic boundary conditions to integrate the equations governing the translational and rotational motions of hydrodynamically interacting particles suspended in the viscous fluid. The first aim of this paper is to examine the behavior of the velocity variance of inertial particles, with small fluctuations produced by Brownian motion at the moderate Péclet numbers. The interplay between mild Brownian forces and hydrodynamic interactions decreases the anisotropy of velocity fluctuations observed in non-Brownian suspensions (Pe1) of sedimenting particles. The simulation results suggest that particle inertia attenuates the magnitude of velocity fluctuations produced by both Brownian motion and viscous hydrodynamic interactions. Additionally, we study the long-time behavior of the velocity fluctuations by calculating their autocorrelation functions and the particle diffusivities. The numerical simulations show clear evidence of particle pressure arising from the flow disturbance produced by hydrodynamic interactions in a suspension. From the particle velocity variance obtained in the present numerical simulations, we propose a simple model for particle-phase pressure as a linear function of the particle volume fraction , which is usually a closure quantity required in models of more complex particulate systems such as fluidized beds.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
7.30
自引率
10.50%
发文量
244
审稿时长
4 months
期刊介绍:
The International Journal of Multiphase Flow publishes analytical, numerical and experimental articles of lasting interest. The scope of the journal includes all aspects of mass, momentum and energy exchange phenomena among different phases such as occur in disperse flows, gas–liquid and liquid–liquid flows, flows in porous media, boiling, granular flows and others.
The journal publishes full papers, brief communications and conference announcements.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


