利用总化学势概括毛细管压力概念,并由此推导出多孔介质中两相流动的控制方程
IF 3.6
2区 工程技术
Q1 MECHANICS
International Journal of Multiphase Flow
Pub Date : 2024-10-11
DOI:10.1016/j.ijmultiphaseflow.2024.105024
引用次数: 0
摘要
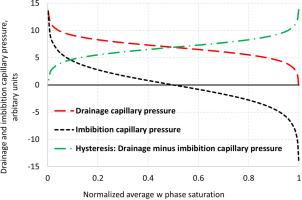
这项研究提出了多孔介质中两相流动的治理方程(GE),它将毛细管压力与摩擦压力损失以及向处于静止或扩散平衡状态的系统提供的外部化学势联系起来。它以非润湿相和润湿相化学势(物理、压力或能量密度)之间的差异为基础,从而概括了毛细管压力的概念。由于流体饱和度的变化,相内部化学势的差异表现为界面面积和熵密度的变化,并由系统外部化学势提供平衡。根据扩散平衡准则,提出了毛细管压力概念的定义。全球定位系统可以解释动态毛细管压力项、滞后的起源,并将注入不同盐度水相时毛细管压力曲线的移动与所有其他化学势作用联系起来。它可以连接、限制并量化所有可以用化学势来表述的效应,因为它是基于所有两相流系统在静止或扩散平衡时必须遵守的平衡方程。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Using the total chemical potential to generalize the capillary pressure concept and therefrom derive a governing equation for two-phase flow in porous media
This work presents a governing equation (GE) for two-phase flow in porous media connecting capillary pressure to frictional pressure loss and external chemical potential supplied to a system in either stationary or diffusive equilibrium. It is based on the difference between non-wetting and wetting phase chemical potential (physically, pressure or energy density), which leads to a generalization of the capillary pressure concept. The difference in phase internal chemical potentials is characterized by changes in both interfacial areas and entropy densities due to variation in fluid saturations and is balanced by the system external chemical potential supplied. A definition of the capillary pressure concept is formulated based on the diffusive equilibrium criterion. The GE can explain the origin of the dynamic capillary pressure term, hysteresis, and connect the shift in the capillary pressure curve upon injecting water phases with varying salinities to all the other chemical potentials acting. It can connect, constrain, and potentially quantify all effects which can be formulated in terms of chemical potentials since it is based on a balance equation all two-phase flow systems must obey when either in stationary or diffusive equilibrium.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
7.30
自引率
10.50%
发文量
244
审稿时长
4 months
期刊介绍:
The International Journal of Multiphase Flow publishes analytical, numerical and experimental articles of lasting interest. The scope of the journal includes all aspects of mass, momentum and energy exchange phenomena among different phases such as occur in disperse flows, gas–liquid and liquid–liquid flows, flows in porous media, boiling, granular flows and others.
The journal publishes full papers, brief communications and conference announcements.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


