薄锥体衍射的理论与实验研究

IF 0.9 4区物理与天体物理 Q4 ACOUSTICS

Acoustical Physics Pub Date : 2024-09-18 DOI:10.1134/S1063771023600754

A. Yu. Laptev, A. I. Korol’kov, A. V. Shanin

{"title":"薄锥体衍射的理论与实验研究","authors":"A. Yu. Laptev, A. I. Korol’kov, A. V. Shanin","doi":"10.1134/S1063771023600754","DOIUrl":null,"url":null,"abstract":"<p>The problem of diffraction of ultrasonic waves by a sharp-angled rigid cone is studied. In the framework of the parabolic equation method, an analytical solution of the problem with an arbitrarily located point source is constructed. Namely, the problem is reduced to the Volterra boundary integral equation, which can be solved using the Fourier transform. An experimental measurement of the diffracted field is performed. The experiment is based on the M-sequence method adapted for narrowband sound sources. The experimental and theoretical results are compared.</p>","PeriodicalId":455,"journal":{"name":"Acoustical Physics","volume":"70 3","pages":"424 - 433"},"PeriodicalIF":0.9000,"publicationDate":"2024-09-18","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Theoretical and Experimental Study of Diffraction by a Thin Cone\",\"authors\":\"A. Yu. Laptev, A. I. Korol’kov, A. V. Shanin\",\"doi\":\"10.1134/S1063771023600754\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<p>The problem of diffraction of ultrasonic waves by a sharp-angled rigid cone is studied. In the framework of the parabolic equation method, an analytical solution of the problem with an arbitrarily located point source is constructed. Namely, the problem is reduced to the Volterra boundary integral equation, which can be solved using the Fourier transform. An experimental measurement of the diffracted field is performed. The experiment is based on the M-sequence method adapted for narrowband sound sources. The experimental and theoretical results are compared.</p>\",\"PeriodicalId\":455,\"journal\":{\"name\":\"Acoustical Physics\",\"volume\":\"70 3\",\"pages\":\"424 - 433\"},\"PeriodicalIF\":0.9000,\"publicationDate\":\"2024-09-18\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Acoustical Physics\",\"FirstCategoryId\":\"101\",\"ListUrlMain\":\"https://link.springer.com/article/10.1134/S1063771023600754\",\"RegionNum\":4,\"RegionCategory\":\"物理与天体物理\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q4\",\"JCRName\":\"ACOUSTICS\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Acoustical Physics","FirstCategoryId":"101","ListUrlMain":"https://link.springer.com/article/10.1134/S1063771023600754","RegionNum":4,"RegionCategory":"物理与天体物理","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q4","JCRName":"ACOUSTICS","Score":null,"Total":0}

引用次数: 0

摘要

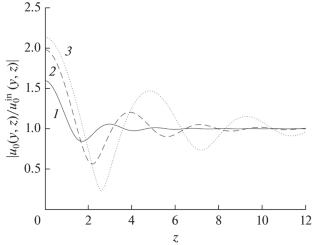
摘要研究了锐角刚性锥对超声波的衍射问题。在抛物线方程方法的框架下，构建了任意位置点源问题的解析解。也就是说，该问题被简化为 Volterra 边界积分方程，可以使用傅立叶变换来求解。对衍射场进行了实验测量。实验基于适用于窄带声源的 M 序列法。实验结果与理论结果进行了比较。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

$Theoretical and Experimental Study of Diffraction by a Thin Cone$

查看原文本刊更多论文

Theoretical and Experimental Study of Diffraction by a Thin Cone

The problem of diffraction of ultrasonic waves by a sharp-angled rigid cone is studied. In the framework of the parabolic equation method, an analytical solution of the problem with an arbitrarily located point source is constructed. Namely, the problem is reduced to the Volterra boundary integral equation, which can be solved using the Fourier transform. An experimental measurement of the diffracted field is performed. The experiment is based on the M-sequence method adapted for narrowband sound sources. The experimental and theoretical results are compared.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Acoustical Physics 物理-声学

CiteScore

1.60

自引率

50.00%

发文量

审稿时长

3.5 months

期刊介绍： Acoustical Physics is an international peer reviewed journal published with the participation of the Russian Academy of Sciences. It covers theoretical and experimental aspects of basic and applied acoustics: classical problems of linear acoustics and wave theory; nonlinear acoustics; physical acoustics; ocean acoustics and hydroacoustics; atmospheric and aeroacoustics; acoustics of structurally inhomogeneous solids; geological acoustics; acoustical ecology, noise and vibration; chamber acoustics, musical acoustics; acoustic signals processing, computer simulations; acoustics of living systems, biomedical acoustics; physical principles of engineering acoustics. The journal publishes critical reviews, original articles, short communications, and letters to the editor. It covers theoretical and experimental aspects of basic and applied acoustics. The journal welcomes manuscripts from all countries in the English or Russian language.