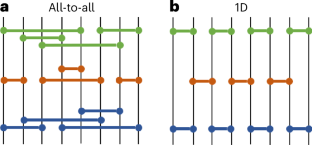
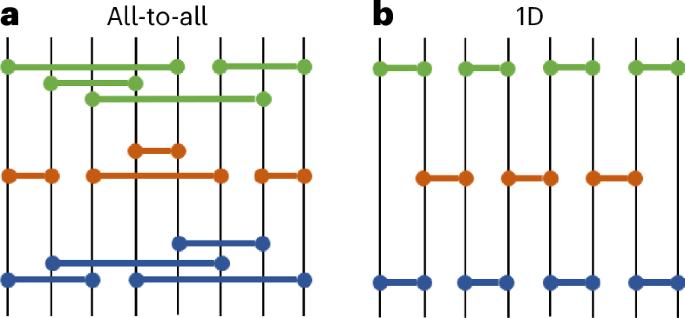
Complexity and order in approximate quantum error-correcting codes

Some form of quantum error correction is necessary to produce large-scale fault-tolerant quantum computers and finds broad relevance in physics. Most studies customarily assume exact correction. However, codes that may only enable approximate quantum error correction (AQEC) could be useful and intrinsically important in many practical and physical contexts. Here we establish rigorous connections between quantum circuit complexity and AQEC capability. Our analysis covers systems with both all-to-all connectivity and geometric scenarios like lattice systems. To this end, we introduce a type of code parameter that we call subsystem variance, which is closely related to the optimal AQEC precision. For a code encoding k logical qubits in n physical qubits, we find that if the subsystem variance is below an O(k/n) threshold, then any state in the code subspace must obey certain circuit complexity lower bounds, which identify non-trivial phases of codes. This theory of AQEC provides a versatile framework for understanding quantum complexity and order in many-body quantum systems, generating new insights for wide-ranging important physical scenarios such as topological order and critical quantum systems. Our results suggest that O(1/n) represents a common, physically profound scaling threshold of subsystem variance for features associated with non-trivial quantum order. Approximate—rather than exact—quantum error correction is a useful but relatively unexplored idea in quantum computing and many-body physics. A theoretical framework has now been established based on connections with quantum circuit complexity.