机械系统的时空对称性和非互易参数共振
IF 2.4
3区 物理与天体物理
Q1 Mathematics
引用次数: 0
摘要
由于参数共振现象,具有时间调制参数的线性机械系统会产生振幅随时间呈指数增长或衰减的振荡。虽然单个振荡器的共振特性已广为人知,但耦合振荡器系统的共振特性仍难以确定。在这里,我们利用经典力学的实值和对称性质所产生的内部对称性,确定了时间调制机械系统的参数共振条件。我们还确定了当系统表现出外部对称性时,这些条件如何进一步受到限制。特别是,我们分析了具有时空对称性的系统,在这些系统中,经过空间和时间的离散平移组合后,系统仍保持不变。对于这类系统,我们确定了一个组合时空平移算子,它能提供比 Floquet 算子更多的系统动力学信息,并利用它推导出行波单向放大的条件。我们基于对称性的精确理论框架能够设计出奇特的响应,如动态机械超材料中的非互惠传输和单向放大,并可推广到所有遵守时空对称性的物理系统。本文章由计算机程序翻译,如有差异,请以英文原文为准。
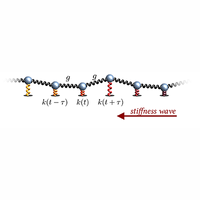
Space-time symmetry and nonreciprocal parametric resonance in mechanical systems
Linear mechanical systems with time-modulated parameters can harbor oscillations with amplitudes that grow or decay exponentially with time due to the phenomenon of parametric resonance. While the resonance properties of individual oscillators are well understood, those of systems of coupled oscillators remain challenging to characterize. Here we determine the parametric resonance conditions for time-modulated mechanical systems by exploiting the internal symmetries arising from the real-valued and symplectic nature of classical mechanics. We also determine how these conditions are further constrained when the system exhibits external symmetries. In particular, we analyze systems with space-time symmetry where the system remains invariant after a combination of discrete translation in both space and time. For such systems, we identify a combined space-time translation operator that provides more information about the dynamics of the system than the Floquet operator does and use it to derive conditions for one-way amplification of traveling waves. Our exact theoretical framework based on symmetries enables the design of exotic responses such as nonreciprocal transport and one-way amplification in dynamic mechanical metamaterials and is generalizable to all physical systems that obey space-time symmetry.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Physical review. E
物理-物理:流体与等离子体
CiteScore
4.60
自引率
16.70%
发文量
0
审稿时长
3.3 months
期刊介绍:
Physical Review E (PRE), broad and interdisciplinary in scope, focuses on collective phenomena of many-body systems, with statistical physics and nonlinear dynamics as the central themes of the journal. Physical Review E publishes recent developments in biological and soft matter physics including granular materials, colloids, complex fluids, liquid crystals, and polymers. The journal covers fluid dynamics and plasma physics and includes sections on computational and interdisciplinary physics, for example, complex networks.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


