论有限生成交换准域的最小配线生成集
IF 0.6
4区 数学
Q3 MATHEMATICS
引用次数: 0
摘要
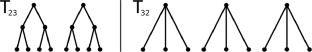
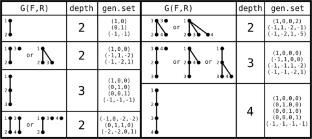
我们研究了理想简单交换半域,并总结了它们的分类结果,特别是当它们是有限生成时。在(副)半场的主要情况下,我们考虑它们的最小生成数,并证明它与相关根森林的深度呈线性增长。本文章由计算机程序翻译,如有差异,请以英文原文为准。
On minimal semiring generating sets of finitely generated commutative parasemifields
We study ideal-simple commutative semirings and summarize the results giving their classification, in particular when they are finitely generated. In the principal case of (para)semifields, we then consider their minimal number of generators and show that it grows linearly with the depth of an associated rooted forest.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Algebra Universalis
数学-数学
CiteScore
1.00
自引率
16.70%
发文量
34
审稿时长
3 months
期刊介绍:
Algebra Universalis publishes papers in universal algebra, lattice theory, and related fields. In a pragmatic way, one could define the areas of interest of the journal as the union of the areas of interest of the members of the Editorial Board. In addition to research papers, we are also interested in publishing high quality survey articles.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


