完美电导体平面内任意方位半椭圆形空腔的散射

IF 1.1 4区计算机科学 Q4 ENGINEERING, ELECTRICAL & ELECTRONIC

Iet Microwaves Antennas & Propagation Pub Date : 2024-03-06 DOI:10.1049/mia2.12466

Mehdi Bozorgi

{"title":"完美电导体平面内任意方位半椭圆形空腔的散射","authors":"Mehdi Bozorgi","doi":"10.1049/mia2.12466","DOIUrl":null,"url":null,"abstract":"<p>A semi-analytic solution for scattering from a semi-elliptical cavity with arbitrary orientation placed in a perfect electric conductor plane is suggested. By imposing the boundary conditions at an elliptical border, three independent equations containing the Mathieu functions series are extracted. Finally, the solution of the problem is reduced to the solution of a simple system of linear equations by using Mathieu function addition theorem and the orthogonal properties of the angular Mathieu functions. Some examples are presented to demonstrate the reliability and the accuracy of the proposed solution.</p>","PeriodicalId":13374,"journal":{"name":"Iet Microwaves Antennas & Propagation","volume":"18 5","pages":"382-390"},"PeriodicalIF":1.1000,"publicationDate":"2024-03-06","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"https://onlinelibrary.wiley.com/doi/epdf/10.1049/mia2.12466","citationCount":"0","resultStr":"{\"title\":\"Scattering from a semi-elliptical cavity with arbitrary orientation placed in a perfect electric conductor plane\",\"authors\":\"Mehdi Bozorgi\",\"doi\":\"10.1049/mia2.12466\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<p>A semi-analytic solution for scattering from a semi-elliptical cavity with arbitrary orientation placed in a perfect electric conductor plane is suggested. By imposing the boundary conditions at an elliptical border, three independent equations containing the Mathieu functions series are extracted. Finally, the solution of the problem is reduced to the solution of a simple system of linear equations by using Mathieu function addition theorem and the orthogonal properties of the angular Mathieu functions. Some examples are presented to demonstrate the reliability and the accuracy of the proposed solution.</p>\",\"PeriodicalId\":13374,\"journal\":{\"name\":\"Iet Microwaves Antennas & Propagation\",\"volume\":\"18 5\",\"pages\":\"382-390\"},\"PeriodicalIF\":1.1000,\"publicationDate\":\"2024-03-06\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"https://onlinelibrary.wiley.com/doi/epdf/10.1049/mia2.12466\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Iet Microwaves Antennas & Propagation\",\"FirstCategoryId\":\"94\",\"ListUrlMain\":\"https://onlinelibrary.wiley.com/doi/10.1049/mia2.12466\",\"RegionNum\":4,\"RegionCategory\":\"计算机科学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q4\",\"JCRName\":\"ENGINEERING, ELECTRICAL & ELECTRONIC\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Iet Microwaves Antennas & Propagation","FirstCategoryId":"94","ListUrlMain":"https://onlinelibrary.wiley.com/doi/10.1049/mia2.12466","RegionNum":4,"RegionCategory":"计算机科学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q4","JCRName":"ENGINEERING, ELECTRICAL & ELECTRONIC","Score":null,"Total":0}

引用次数: 0

摘要

提出了完美电导体平面内任意方位半椭圆形空腔散射的半解析解法。通过在椭圆边界施加边界条件，提取出包含马修函数序列的三个独立方程。最后，利用马修函数加法定理和角马修函数的正交特性，将问题的求解简化为一个简单线性方程组的求解。本文列举了一些示例来证明所提出的解决方案的可靠性和准确性。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

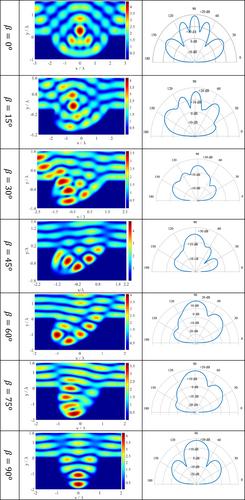
Scattering from a semi-elliptical cavity with arbitrary orientation placed in a perfect electric conductor plane

查看原文本刊更多论文

Scattering from a semi-elliptical cavity with arbitrary orientation placed in a perfect electric conductor plane

A semi-analytic solution for scattering from a semi-elliptical cavity with arbitrary orientation placed in a perfect electric conductor plane is suggested. By imposing the boundary conditions at an elliptical border, three independent equations containing the Mathieu functions series are extracted. Finally, the solution of the problem is reduced to the solution of a simple system of linear equations by using Mathieu function addition theorem and the orthogonal properties of the angular Mathieu functions. Some examples are presented to demonstrate the reliability and the accuracy of the proposed solution.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Iet Microwaves Antennas & Propagation 工程技术-电信学

CiteScore

4.30

自引率

5.90%

发文量

109

审稿时长

7 months

期刊介绍： Topics include, but are not limited to: Microwave circuits including RF, microwave and millimetre-wave amplifiers, oscillators, switches, mixers and other components implemented in monolithic, hybrid, multi-chip module and other technologies. Papers on passive components may describe transmission-line and waveguide components, including filters, multiplexers, resonators, ferrite and garnet devices. For applications, papers can describe microwave sub-systems for use in communications, radar, aerospace, instrumentation, industrial and medical applications. Microwave linear and non-linear measurement techniques. Antenna topics including designed and prototyped antennas for operation at all frequencies; multiband antennas, antenna measurement techniques and systems, antenna analysis and design, aperture antenna arrays, adaptive antennas, printed and wire antennas, microstrip, reconfigurable, conformal and integrated antennas. Computational electromagnetics and synthesis of antenna structures including phased arrays and antenna design algorithms. Radiowave propagation at all frequencies and environments. Current Special Issue. Call for papers: Metrology for 5G Technologies - https://digital-library.theiet.org/files/IET_MAP_CFP_M5GT_SI2.pdf