具有对称结构的有理矩阵函数的结构化特征值后向误差

IF 16.4 1区化学 Q1 CHEMISTRY, MULTIDISCIPLINARY

Accounts of Chemical Research Pub Date : 2024-02-17 DOI:10.1007/s10543-024-01010-3

Anshul Prajapati, Punit Sharma

引用次数: 0

摘要

当把复数 \(\lambda \)视为带有对称结构的有理矩阵函数的近似特征值时，我们推导出复数 \(\lambda \)的结构后向误差的可计算公式。我们考虑了对称结构、偏对称结构、赫米特结构、偏赫米特结构、（*）-palindromic结构、T-even结构、T-odd结构、（*）-even结构和（*）-odd结构。数值实验表明，保结构和任意扰动的后向误差有很大不同。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

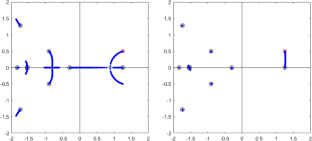
Structured eigenvalue backward errors for rational matrix functions with symmetry structures

查看原文本刊更多论文

Structured eigenvalue backward errors for rational matrix functions with symmetry structures

We derive computable formulas for the structured backward errors of a complex number \(\lambda \) when considered as an approximate eigenvalue of rational matrix functions that carry a symmetry structure. We consider symmetric, skew-symmetric, Hermitian, skew-Hermitian, \(*\)-palindromic, T-even, T-odd, \(*\)-even, and \(*\)-odd structures. Numerical experiments show that the backward errors with respect to structure-preserving and arbitrary perturbations are significantly different.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Accounts of Chemical Research 化学-化学综合

CiteScore

31.40

自引率

1.10%

发文量

312

审稿时长

2 months

期刊介绍： Accounts of Chemical Research presents short, concise and critical articles offering easy-to-read overviews of basic research and applications in all areas of chemistry and biochemistry. These short reviews focus on research from the author’s own laboratory and are designed to teach the reader about a research project. In addition, Accounts of Chemical Research publishes commentaries that give an informed opinion on a current research problem. Special Issues online are devoted to a single topic of unusual activity and significance. Accounts of Chemical Research replaces the traditional article abstract with an article "Conspectus." These entries synopsize the research affording the reader a closer look at the content and significance of an article. Through this provision of a more detailed description of the article contents, the Conspectus enhances the article's discoverability by search engines and the exposure for the research.