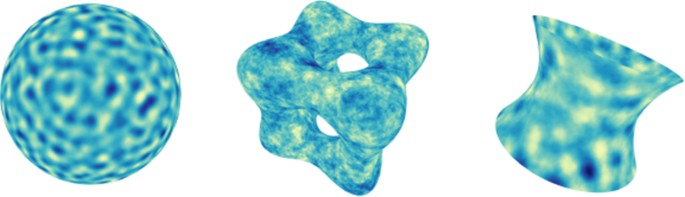
Galerkin–Chebyshev approximation of Gaussian random fields on compact Riemannian manifolds

Abstract A new numerical approximation method for a class of Gaussian random fields on compact connected oriented Riemannian manifolds is introduced. This class of random fields is characterized by the Laplace–Beltrami operator on the manifold. A Galerkin approximation is combined with a polynomial approximation using Chebyshev series. This so-called Galerkin–Chebyshev approximation scheme yields efficient and generic sampling algorithms for Gaussian random fields on manifolds. Strong and weak orders of convergence for the Galerkin approximation and strong convergence orders for the Galerkin–Chebyshev approximation are shown and confirmed through numerical experiments.