带内野的Leslie-Gower捕食-食饵三元模型动力学研究
IF 1.1
4区 数学
Q1 MATHEMATICS
引用次数: 0
摘要
摘要本文研究了一个捕食者-食饵模型,该模型描述了捕食者、中捕食者和顶级捕食者这三个相互作用的物种之间的进化过程,旨在建立一个完整的食物网模型。特别地,分析了解的长期行为,证明了吸收集的存在性,并进行了共存平衡的线性和非线性稳定性分析。本文章由计算机程序翻译,如有差异,请以英文原文为准。
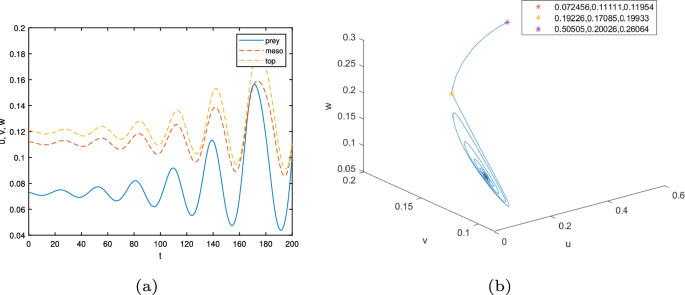
On the dynamics of a Leslie–Gower predator–prey ternary model with intraguild
Abstract In this paper, a predator–prey model with intraguild predation describing the evolution between three interacting species—namely prey, mesopredator and top predator—is investigated, with the aim to model a complete food web. In particular, the longtime behaviour of the solutions is analysed, proving the existence of an absorbing set, and the linear and nonlinear stability analyses of the coexistence equilibrium are performed.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊

Ricerche di Matematica
Mathematics-Applied Mathematics
CiteScore
3.00
自引率
8.30%
发文量
61
期刊介绍:
“Ricerche di Matematica” publishes high-quality research articles in any field of pure and applied mathematics. Articles must be original and written in English. Details about article submission can be found online.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


