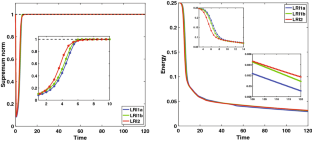
Low regularity integrators for semilinear parabolic equations with maximum bound principles

This paper is concerned with conditionally structure-preserving, low regularity time integration methods for a class of semilinear parabolic equations of Allen–Cahn type. Important properties of such equations include maximum bound principle (MBP) and energy dissipation law; for the former, that means the absolute value of the solution is pointwisely bounded for all the time by some constant imposed by appropriate initial and boundary conditions. The model equation is first discretized in space by the central finite difference, then by iteratively using Duhamel’s formula, first- and second-order low regularity integrators (LRIs) are constructed for time discretization of the semi-discrete system. The proposed LRI schemes are proved to preserve the MBP and the energy stability in the discrete sense. Furthermore, their temporal error estimates are also successfully derived under a low regularity requirement that the exact solution of the semi-discrete problem is only assumed to be continuous in time. Numerical results show that the proposed LRI schemes are more accurate and have better convergence rates than classic exponential time differencing schemes, especially when the interfacial parameter approaches zero.