Artur Alho, Vitor Bessa, Filipe C. Mena
求助PDF
{"title":"相互作用的单标量场势和完美流体动力学","authors":"Artur Alho, Vitor Bessa, Filipe C. Mena","doi":"10.1007/s10884-023-10318-7","DOIUrl":null,"url":null,"abstract":"Abstract Motivated by cosmological models of the early universe we analyse the dynamics of the Einstein equations with a minimally coupled scalar field with monomial potentials $$V(\\phi )=\\frac{(\\lambda \\phi )^{2n}}{2n}$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:mi>V</mml:mi> <mml:mrow> <mml:mo>(</mml:mo> <mml:mi>ϕ</mml:mi> <mml:mo>)</mml:mo> </mml:mrow> <mml:mo>=</mml:mo> <mml:mfrac> <mml:msup> <mml:mrow> <mml:mo>(</mml:mo> <mml:mi>λ</mml:mi> <mml:mi>ϕ</mml:mi> <mml:mo>)</mml:mo> </mml:mrow> <mml:mrow> <mml:mn>2</mml:mn> <mml:mi>n</mml:mi> </mml:mrow> </mml:msup> <mml:mrow> <mml:mn>2</mml:mn> <mml:mi>n</mml:mi> </mml:mrow> </mml:mfrac> </mml:mrow> </mml:math> , $$\\lambda >0$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:mi>λ</mml:mi> <mml:mo>></mml:mo> <mml:mn>0</mml:mn> </mml:mrow> </mml:math> , $$n\\in {\\mathbb {N}}$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:mi>n</mml:mi> <mml:mo>∈</mml:mo> <mml:mi>N</mml:mi> </mml:mrow> </mml:math> , interacting with a perfect fluid with linear equation of state $$p_{\\textrm{pf}}=(\\gamma _{\\textrm{pf}}-1)\\rho _{\\textrm{pf}}$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:msub> <mml:mi>p</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> <mml:mo>=</mml:mo> <mml:mrow> <mml:mo>(</mml:mo> <mml:msub> <mml:mi>γ</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> <mml:mo>-</mml:mo> <mml:mn>1</mml:mn> <mml:mo>)</mml:mo> </mml:mrow> <mml:msub> <mml:mi>ρ</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> </mml:mrow> </mml:math> , $$\\gamma _{\\textrm{pf}}\\in (0,2)$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:msub> <mml:mi>γ</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> <mml:mo>∈</mml:mo> <mml:mrow> <mml:mo>(</mml:mo> <mml:mn>0</mml:mn> <mml:mo>,</mml:mo> <mml:mn>2</mml:mn> <mml:mo>)</mml:mo> </mml:mrow> </mml:mrow> </mml:math> , in flat Robertson–Walker spacetimes. The interaction is a friction-like term of the form $$\\Gamma (\\phi )=\\mu \\phi ^{2p}$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:mi>Γ</mml:mi> <mml:mrow> <mml:mo>(</mml:mo> <mml:mi>ϕ</mml:mi> <mml:mo>)</mml:mo> </mml:mrow> <mml:mo>=</mml:mo> <mml:mi>μ</mml:mi> <mml:msup> <mml:mi>ϕ</mml:mi> <mml:mrow> <mml:mn>2</mml:mn> <mml:mi>p</mml:mi> </mml:mrow> </mml:msup> </mml:mrow> </mml:math> , $$\\mu >0$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:mi>μ</mml:mi> <mml:mo>></mml:mo> <mml:mn>0</mml:mn> </mml:mrow> </mml:math> , $$p\\in {\\mathbb {N}}\\cup \\{0\\}$$ <mml:math xmlns:mml=\"http://www.w3.org/1998/Math/MathML\"> <mml:mrow> <mml:mi>p</mml:mi> <mml:mo>∈</mml:mo> <mml:mi>N</mml:mi> <mml:mo>∪</mml:mo> <mml:mo>{</mml:mo> <mml:mn>0</mml:mn> <mml:mo>}</mml:mo> </mml:mrow> </mml:math> . The analysis relies on the introduction of a new regular 3-dimensional dynamical systems’ formulation of the Einstein equations on a compact state space, and the use of dynamical systems’ tools such as quasi-homogeneous blow-ups and averaging methods involving a time-dependent perturbation parameter.","PeriodicalId":15624,"journal":{"name":"Journal of Dynamics and Differential Equations","volume":"1 1","pages":"0"},"PeriodicalIF":1.3000,"publicationDate":"2023-11-06","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"1","resultStr":"{\"title\":\"Dynamics of Interacting Monomial Scalar Field Potentials and Perfect Fluids\",\"authors\":\"Artur Alho, Vitor Bessa, Filipe C. Mena\",\"doi\":\"10.1007/s10884-023-10318-7\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"Abstract Motivated by cosmological models of the early universe we analyse the dynamics of the Einstein equations with a minimally coupled scalar field with monomial potentials $$V(\\\\phi )=\\\\frac{(\\\\lambda \\\\phi )^{2n}}{2n}$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:mi>V</mml:mi> <mml:mrow> <mml:mo>(</mml:mo> <mml:mi>ϕ</mml:mi> <mml:mo>)</mml:mo> </mml:mrow> <mml:mo>=</mml:mo> <mml:mfrac> <mml:msup> <mml:mrow> <mml:mo>(</mml:mo> <mml:mi>λ</mml:mi> <mml:mi>ϕ</mml:mi> <mml:mo>)</mml:mo> </mml:mrow> <mml:mrow> <mml:mn>2</mml:mn> <mml:mi>n</mml:mi> </mml:mrow> </mml:msup> <mml:mrow> <mml:mn>2</mml:mn> <mml:mi>n</mml:mi> </mml:mrow> </mml:mfrac> </mml:mrow> </mml:math> , $$\\\\lambda >0$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:mi>λ</mml:mi> <mml:mo>></mml:mo> <mml:mn>0</mml:mn> </mml:mrow> </mml:math> , $$n\\\\in {\\\\mathbb {N}}$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:mi>n</mml:mi> <mml:mo>∈</mml:mo> <mml:mi>N</mml:mi> </mml:mrow> </mml:math> , interacting with a perfect fluid with linear equation of state $$p_{\\\\textrm{pf}}=(\\\\gamma _{\\\\textrm{pf}}-1)\\\\rho _{\\\\textrm{pf}}$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:msub> <mml:mi>p</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> <mml:mo>=</mml:mo> <mml:mrow> <mml:mo>(</mml:mo> <mml:msub> <mml:mi>γ</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> <mml:mo>-</mml:mo> <mml:mn>1</mml:mn> <mml:mo>)</mml:mo> </mml:mrow> <mml:msub> <mml:mi>ρ</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> </mml:mrow> </mml:math> , $$\\\\gamma _{\\\\textrm{pf}}\\\\in (0,2)$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:msub> <mml:mi>γ</mml:mi> <mml:mtext>pf</mml:mtext> </mml:msub> <mml:mo>∈</mml:mo> <mml:mrow> <mml:mo>(</mml:mo> <mml:mn>0</mml:mn> <mml:mo>,</mml:mo> <mml:mn>2</mml:mn> <mml:mo>)</mml:mo> </mml:mrow> </mml:mrow> </mml:math> , in flat Robertson–Walker spacetimes. The interaction is a friction-like term of the form $$\\\\Gamma (\\\\phi )=\\\\mu \\\\phi ^{2p}$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:mi>Γ</mml:mi> <mml:mrow> <mml:mo>(</mml:mo> <mml:mi>ϕ</mml:mi> <mml:mo>)</mml:mo> </mml:mrow> <mml:mo>=</mml:mo> <mml:mi>μ</mml:mi> <mml:msup> <mml:mi>ϕ</mml:mi> <mml:mrow> <mml:mn>2</mml:mn> <mml:mi>p</mml:mi> </mml:mrow> </mml:msup> </mml:mrow> </mml:math> , $$\\\\mu >0$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:mi>μ</mml:mi> <mml:mo>></mml:mo> <mml:mn>0</mml:mn> </mml:mrow> </mml:math> , $$p\\\\in {\\\\mathbb {N}}\\\\cup \\\\{0\\\\}$$ <mml:math xmlns:mml=\\\"http://www.w3.org/1998/Math/MathML\\\"> <mml:mrow> <mml:mi>p</mml:mi> <mml:mo>∈</mml:mo> <mml:mi>N</mml:mi> <mml:mo>∪</mml:mo> <mml:mo>{</mml:mo> <mml:mn>0</mml:mn> <mml:mo>}</mml:mo> </mml:mrow> </mml:math> . The analysis relies on the introduction of a new regular 3-dimensional dynamical systems’ formulation of the Einstein equations on a compact state space, and the use of dynamical systems’ tools such as quasi-homogeneous blow-ups and averaging methods involving a time-dependent perturbation parameter.\",\"PeriodicalId\":15624,\"journal\":{\"name\":\"Journal of Dynamics and Differential Equations\",\"volume\":\"1 1\",\"pages\":\"0\"},\"PeriodicalIF\":1.3000,\"publicationDate\":\"2023-11-06\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"1\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Journal of Dynamics and Differential Equations\",\"FirstCategoryId\":\"1085\",\"ListUrlMain\":\"https://doi.org/10.1007/s10884-023-10318-7\",\"RegionNum\":4,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q1\",\"JCRName\":\"MATHEMATICS\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Journal of Dynamics and Differential Equations","FirstCategoryId":"1085","ListUrlMain":"https://doi.org/10.1007/s10884-023-10318-7","RegionNum":4,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q1","JCRName":"MATHEMATICS","Score":null,"Total":0}
引用次数: 1
引用
批量引用
Dynamics of Interacting Monomial Scalar Field Potentials and Perfect Fluids
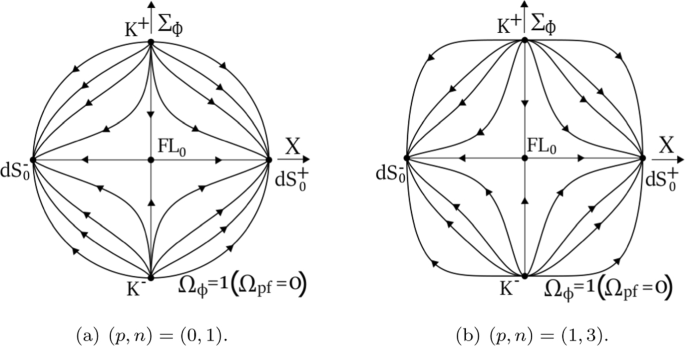
Abstract Motivated by cosmological models of the early universe we analyse the dynamics of the Einstein equations with a minimally coupled scalar field with monomial potentials $$V(\phi )=\frac{(\lambda \phi )^{2n}}{2n}$$ V ( ϕ ) = ( λ ϕ ) 2 n 2 n , $$\lambda >0$$ λ > 0 , $$n\in {\mathbb {N}}$$ n ∈ N , interacting with a perfect fluid with linear equation of state $$p_{\textrm{pf}}=(\gamma _{\textrm{pf}}-1)\rho _{\textrm{pf}}$$ p pf = ( γ pf - 1 ) ρ pf , $$\gamma _{\textrm{pf}}\in (0,2)$$ γ pf ∈ ( 0 , 2 ) , in flat Robertson–Walker spacetimes. The interaction is a friction-like term of the form $$\Gamma (\phi )=\mu \phi ^{2p}$$ Γ ( ϕ ) = μ ϕ 2 p , $$\mu >0$$ μ > 0 , $$p\in {\mathbb {N}}\cup \{0\}$$ p ∈ N ∪ { 0 } . The analysis relies on the introduction of a new regular 3-dimensional dynamical systems’ formulation of the Einstein equations on a compact state space, and the use of dynamical systems’ tools such as quasi-homogeneous blow-ups and averaging methods involving a time-dependent perturbation parameter.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


