大量子位系统希尔伯特空间中的连续渗透
IF 2.3
3区 物理与天体物理
Q2 PHYSICS, MULTIDISCIPLINARY
引用次数: 0
摘要
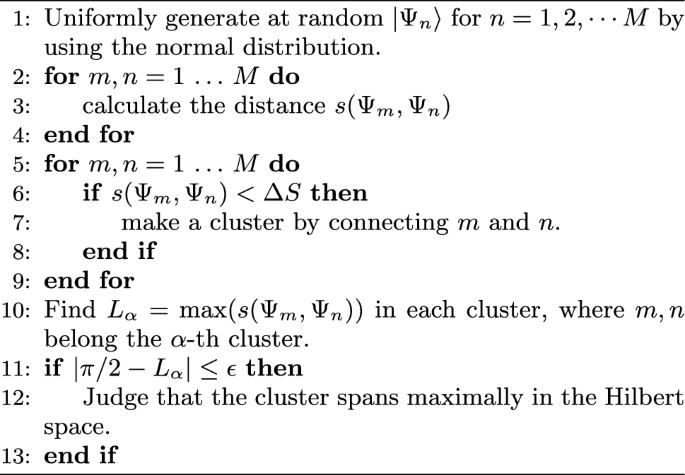
渗透理论的发展是由其在各个科学分支,特别是在统计物理学中的大量应用而形成的,并且主要局限于欧几里得空间的情况。它的中心概念之一,渗透跃迁,是通过无限簇的出现来定义的,因此不能用于紧致空间,例如N -量子比特系统的希尔伯特空间。本文对超球覆盖的随机空间进行了推广,引入了“极大簇”的概念。我们的数值计算再现了超球半径和覆盖密度之间的标准幂律关系,但表明随着量子比特数量的增加,指数会迅速消失(即,希尔伯特空间维度的指数增长使得有限尺寸超球的覆盖效率低下)。因此,与希尔伯特空间中的随机游走模型相比,渗透跃迁不是多量子位系统行为的有效模型。然而,我们对紧度量空间中的渗透过渡的方法可能证明对其在其他情况下的严格处理是有用的。本文章由计算机程序翻译,如有差异,请以英文原文为准。
Continuous percolation in a Hilbert space for a large system of qubits
Abstract The development of percolation theory was historically shaped by its numerous applications in various branches of science, in particular in statistical physics, and was mainly constrained to the case of Euclidean spaces. One of its central concepts, the percolation transition, is defined through the appearance of the infinite cluster, and therefore cannot be used in compact spaces, such as the Hilbert space of an N -qubit system. Here, we propose its generalization for the case of a random space covering by hyperspheres, introducing the concept of a “maximal cluster”. Our numerical calculations reproduce the standard power-law relation between the hypersphere radius and the cover density, but show that as the number of qubits increases, the exponent quickly vanishes (i.e., the exponentially increasing dimensionality of the Hilbert space makes its covering by finite-size hyperspheres inefficient). Therefore the percolation transition is not an efficient model for the behavior of multiqubit systems, compared to the random walk model in the Hilbert space. However, our approach to the percolation transition in compact metric spaces may prove useful for its rigorous treatment in other contexts.
求助全文
通过发布文献求助,成功后即可免费获取论文全文。
去求助
来源期刊
CiteScore
5.10
自引率
10.70%
发文量
313
审稿时长
3-8 weeks
期刊介绍:
EPJ - Special Topics (EPJ ST) publishes topical issues which are collections of review-type articles or extensive, detailed progress reports. Each issue is focused on a specific subject matter of topical interest.
The journal scope covers the whole spectrum of pure and applied physics, including related subjects such as Materials Science, Physical Biology, Physical Chemistry, and Complex Systems with particular emphasis on interdisciplinary topics in physics and related fields.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


