维诺格拉多夫中值定理旧论点的解耦解释

IF 0.8 3区数学 Q2 MATHEMATICS

Mathematika Pub Date : 2023-11-12 DOI:10.1112/mtk.12231

Brian Cook, Kevin Hughes, Zane Kun Li, Akshat Mudgal, Olivier Robert, Po-Lam Yung

引用次数: 2

摘要

我们将Karatsuba在1973年关于维诺格拉多夫中值定理的论证的改进解释为解耦语言。我们讨论的主要目标是回答维诺格拉多夫中值定理的旧部分进展中的精确解计数在傅里叶解耦理论中对应的是什么。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

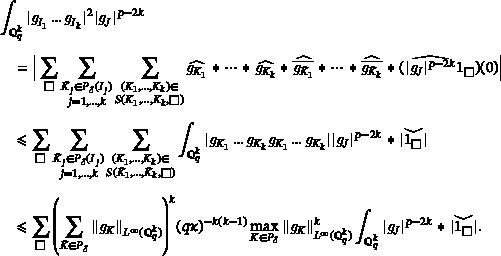
A decoupling interpretation of an old argument for Vinogradov's Mean Value Theorem

查看原文本刊更多论文

A decoupling interpretation of an old argument for Vinogradov's Mean Value Theorem

We interpret into decoupling language a refinement of a 1973 argument due to Karatsuba on Vinogradov's mean value theorem. The main goal of our argument is to answer what precisely solution counting in older partial progress on Vinogradov's mean value theorem corresponds to in Fourier decoupling theory.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Mathematika MATHEMATICS, APPLIED-MATHEMATICS

CiteScore

1.40

自引率

0.00%

发文量

审稿时长

>12 weeks

期刊介绍： Mathematika publishes both pure and applied mathematical articles and has done so continuously since its founding by Harold Davenport in the 1950s. The traditional emphasis has been towards the purer side of mathematics but applied mathematics and articles addressing both aspects are equally welcome. The journal is published by the London Mathematical Society, on behalf of its owner University College London, and will continue to publish research papers of the highest mathematical quality.