Non-Hermitian topological phase transitions controlled by nonlinearity
IF 17.6
1区 物理与天体物理
Q1 PHYSICS, MULTIDISCIPLINARY
引用次数: 0
Abstract
Manipulating topological invariants is possible by modifying the global properties of optical devices to alter their band structures. This could be achieved by statically altering devices or dynamically reconfiguring devices with considerably different geometric parameters, even though it inhibits switching speed. Recently, optical nonlinearity has emerged as a tool for tailoring topological and non-Hermitian (NH) properties, promising fast manipulation of topological phases. In this work, we observe topologically protected NH phase transitions driven by optical nonlinearity in a silicon nanophotonic Floquet topological insulator. The phase transition occurs from forbidden bandgaps to NH conducting edge modes, which emerge at a nonlinearity-induced gain–loss junction along the boundaries of a topological insulator. We find static NH edge modes and dynamic phase transitions involving exceptional points at a speed of hundreds of picoseconds, which inherently retain topological protections against fabrication imperfections. This work shows an interplay between topology and non-Hermiticity by means of nonlinear optics, and it provides a way of manipulating multiple phase transitions at high speeds that is applicable to many other materials with strong nonlinearities, which could promote the development of unconventionally robust light-controlled devices for classical and quantum applications. The phase transition from a topologically trivial state to non-Hermitian conducting edge modes can be controlled by optical nonlinearities, achieving picosecond switching speeds.
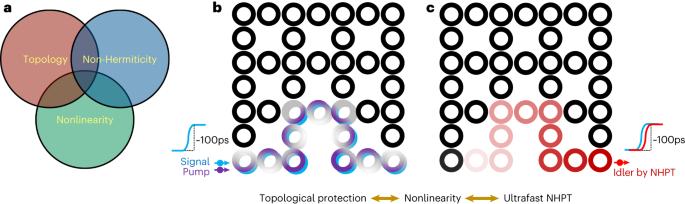
由非线性控制的非赫米拓扑相变
通过修改光学设备的全局属性来改变其带状结构,就有可能操纵拓扑不变性。这可以通过静态改变器件或动态重新配置几何参数差异较大的器件来实现,尽管这会抑制开关速度。最近,光学非线性已成为一种定制拓扑和非赫米提(NH)特性的工具,有望快速操纵拓扑相位。在这项工作中,我们在硅纳米光子 Floquet 拓扑绝缘体中观察到了由光非线性驱动的拓扑保护 NH 相变。相变发生在从禁带隙到 NH 传导边缘模式之间,这种相变出现在拓扑绝缘体边界的非线性诱导增益-损耗交界处。我们发现了静态 NH 边缘模式和涉及例外点的动态相变,其速度可达数百皮秒,本质上保留了拓扑保护功能,防止制造缺陷。这项工作通过非线性光学手段展示了拓扑和非恒定性之间的相互作用,并提供了一种高速操纵多重相变的方法,这种方法适用于许多其他具有强非线性的材料,可促进开发用于经典和量子应用的非传统稳健光控设备。从拓扑琐碎态到非赫米提传导边缘模式的相变可由光非线性控制,实现皮秒级的切换速度。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Nature Physics
物理-物理:综合
CiteScore
30.40
自引率
2.00%
发文量
349
审稿时长
4-8 weeks
期刊介绍:
Nature Physics is dedicated to publishing top-tier original research in physics with a fair and rigorous review process. It provides high visibility and access to a broad readership, maintaining high standards in copy editing and production, ensuring rapid publication, and maintaining independence from academic societies and other vested interests.
The journal presents two main research paper formats: Letters and Articles. Alongside primary research, Nature Physics serves as a central source for valuable information within the physics community through Review Articles, News & Views, Research Highlights covering crucial developments across the physics literature, Commentaries, Book Reviews, and Correspondence.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


