The exactness of the ℓ1 penalty function for a class of mathematical programs with generalized complementarity constraints

Abstract

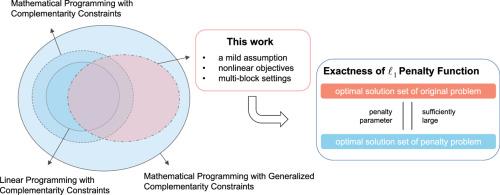
In a mathematical program with generalized complementarity constraints (MPGCC), complementarity relation is imposed between each pair of variable blocks. MPGCC includes the traditional mathematical program with complementarity constraints (MPCC) as a special case. On account of the disjunctive feasible region, MPCC and MPGCC are generally difficult to handle. The ${\ell }_1$ penalty method, often adopted in computation, opens a way of circumventing the difficulty. Yet it remains unclear about the exactness of the ${\ell }_1$ penalty function, namely, whether there exists a sufficiently large penalty parameter so that the penalty problem shares the optimal solution set with the original one. In this paper, we consider a class of MPGCCs that are of multi-affine objective functions. This problem class finds applications in various fields, e.g., the multi-marginal optimal transport problems in many-body quantum physics and the pricing problems in network transportation. We first provide an instance from this class, the exactness of whose ${\ell }_1$ penalty function cannot be derived by existing tools. We then establish the exactness results under rather mild conditions. Our results cover those existing ones for MPCC and apply to multi-block contexts.