A Rate-Reduced Neuron Model for Complex Spiking Behavior.

IF 2.3 4区医学 Q1 Neuroscience

Journal of Mathematical Neuroscience Pub Date : 2017-12-11 DOI:10.1186/s13408-017-0055-3

Koen Dijkstra, Yuri A Kuznetsov, Michel J A M van Putten, Stephan A van Gils

引用次数: 1

Abstract

We present a simple rate-reduced neuron model that captures a wide range of complex, biologically plausible, and physiologically relevant spiking behavior. This includes spike-frequency adaptation, postinhibitory rebound, phasic spiking and accommodation, first-spike latency, and inhibition-induced spiking. Furthermore, the model can mimic different neuronal filter properties. It can be used to extend existing neural field models, adding more biological realism and yielding a richer dynamical structure. The model is based on a slight variation of the Rulkov map.

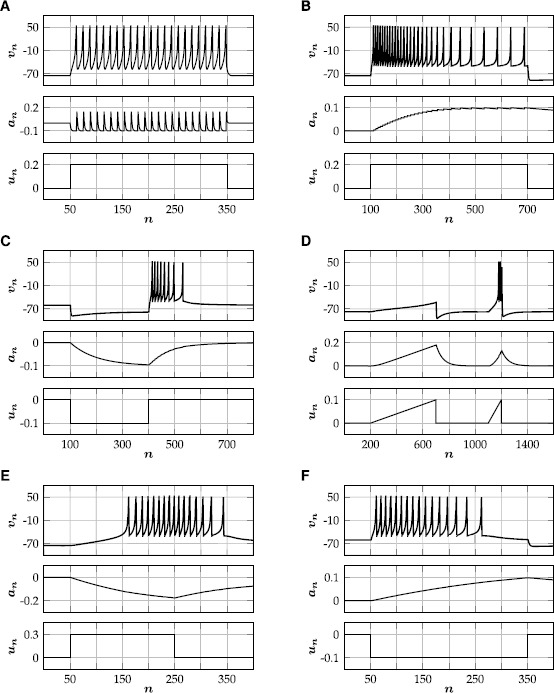
Abstract Image

查看原文本刊更多论文

复杂尖峰行为的速率降低神经元模型。

我们提出了一个简单的速率降低神经元模型，该模型捕获了广泛的复杂的、生物学上合理的和生理上相关的尖峰行为。这包括尖峰频率适应、抑制后反弹、阶段性尖峰和调节、首次尖峰潜伏期和抑制诱导尖峰。此外，该模型可以模拟不同的神经元滤波特性。它可以用于扩展现有的神经场模型，增加更多的生物真实感，并产生更丰富的动态结构。该模型是基于鲁科夫地图的一个细微变化。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Journal of Mathematical Neuroscience Neuroscience-Neuroscience (miscellaneous)

自引率

0.00%

发文量

审稿时长

13 weeks

期刊介绍： The Journal of Mathematical Neuroscience (JMN) publishes research articles on the mathematical modeling and analysis of all areas of neuroscience, i.e., the study of the nervous system and its dysfunctions. The focus is on using mathematics as the primary tool for elucidating the fundamental mechanisms responsible for experimentally observed behaviours in neuroscience at all relevant scales, from the molecular world to that of cognition. The aim is to publish work that uses advanced mathematical techniques to illuminate these questions. It publishes full length original papers, rapid communications and review articles. Papers that combine theoretical results supported by convincing numerical experiments are especially encouraged. Papers that introduce and help develop those new pieces of mathematical theory which are likely to be relevant to future studies of the nervous system in general and the human brain in particular are also welcome.