Soret and Dufour effects in two-phase boundary layer flow of non-Newtonian and Newtonian fluids with uniform shear strength ratio

Abstract

Background

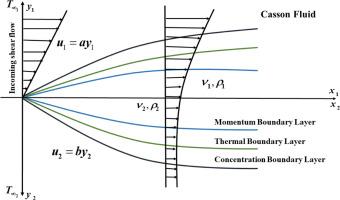
This study numerically investigates heat and mass transfer in two-phase boundary layer shear flows, focusing on a non-Newtonian Casson fluid interacting with an adjacent Newtonian fluid under the influence of thermal radiation. By incorporating both Soret and Dufour effects, we examine how temperature gradients influence mass flux and how concentration differences affect energy flux. These coupled effects are essential for applications involving simultaneous heat and mass transport, such as chemical reactors, separation processes, and thermal management systems.

Methods

The analysis explores boundary layer convergence with varying shear intensities, utilizing the MATLAB solver “bvp4c” with appropriate similarity transformations for computational accuracy. The resulting numerical data are presented in graphical form to illustrate the impact of key parameters on flow characteristics. Additionally, variations in the Sherwood number, Nusselt number, and interfacial shear stress are depicted for each fluid across different parameter values. To evaluate the accuracy of our numerical method, we conducted a comparative analysis with the results reported by Weidman and Wang [4] and Wang [2]. This comparison demonstrated an excellent match, confirming the reliability of our approach, as shown in Table 1 and Fig. 2.

Significant findings

The results demonstrate that the fluid temperature increases with the Dufour number, while the concentration rises with the Soret number. Higher Casson parameter values lead to a reduction in interfacial shear stress in both fluids. Furthermore, the Nusselt and Sherwood numbers increase with an enhanced radiation parameter, highlighting the influence of thermal radiation on heat and mass transfer. These insights offer a valuable understanding of flow behavior in systems with coupled thermal and concentration gradients.