Anderson transition and mobility edges on hyperbolic lattices with randomly connected boundaries
IF 5.8
1区 物理与天体物理
Q1 PHYSICS, MULTIDISCIPLINARY
引用次数: 0
Abstract
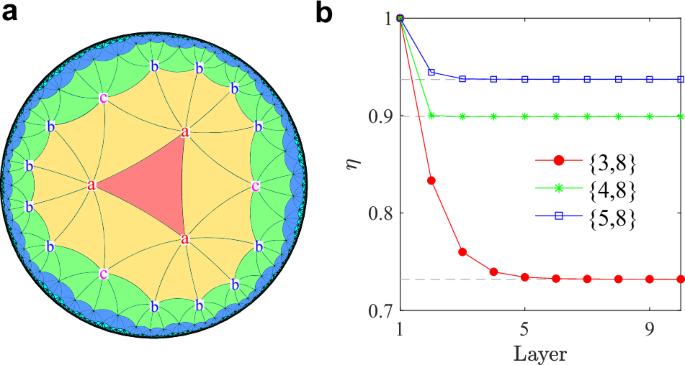
Hyperbolic lattices, formed by tessellating the hyperbolic plane with regular polygons, exhibit a diverse range of exotic physical phenomena beyond conventional Euclidean lattices. Here, we investigate the impact of disorder on hyperbolic lattices and reveal that the Anderson localization occurs at strong disorder strength, accompanied by the presence of mobility edges. Taking the hyperbolic {p, q} = {3, 8} and {p, q} = {4, 8} lattices as examples, we employ finite-size scaling of both spectral statistics and the inverse participation ratio to pinpoint the transition point and critical exponents. Our findings indicate that the transition points tend to increase with larger values of {p, q} or curvature. In the limiting case of {∞, q}, we further determine its Anderson transition using the cavity method, drawing parallels with the random regular graph. Our work lays the cornerstone for a comprehensive understanding of Anderson transition and mobility edges on hyperbolic lattices. Anderson localization is a paradigmatic topic of condensed matter physics used to explain the insulating behavior of materials. This paper investigates the effect of disorder in hyperbolic lattices and finds that Anderson localization occurs at strong disorder strength, accompanied by the presence of mobility edges.
具有随机连接边界的双曲晶格上的安德森转换和流动边缘
双曲晶格是用规则多边形拼成双曲面而形成的,它展现了超越传统欧几里得晶格的各种奇异物理现象。在这里,我们研究了无序对双曲晶格的影响,并揭示了安德森局域化发生在强无序强度下,并伴随着流动边缘的存在。以双曲{p, q} = {3, 8}和{p, q} = {4, 8}晶格为例,我们利用谱统计和反参与比的有限大小缩放来精确定位过渡点和临界指数。我们的研究结果表明,过渡点往往随着{p, q}或曲率值的增大而增大。在{∞, q}的极限情况下,我们使用空穴法进一步确定了安德森过渡,并将其与随机正则图进行了比较。我们的工作为全面理解双曲晶格上的安德森转换和流动边缘奠定了基石。安德森局域化是凝聚态物理学的一个典型课题,用于解释材料的绝缘行为。本文研究了双曲晶格中无序的影响,发现安德森局域化发生在强无序强度下,并伴随着迁移率边缘的存在。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊
Communications Physics
Physics and Astronomy-General Physics and Astronomy
CiteScore
8.40
自引率
3.60%
发文量
276
审稿时长
13 weeks
期刊介绍:
Communications Physics is an open access journal from Nature Research publishing high-quality research, reviews and commentary in all areas of the physical sciences. Research papers published by the journal represent significant advances bringing new insight to a specialized area of research in physics. We also aim to provide a community forum for issues of importance to all physicists, regardless of sub-discipline.
The scope of the journal covers all areas of experimental, applied, fundamental, and interdisciplinary physical sciences. Primary research published in Communications Physics includes novel experimental results, new techniques or computational methods that may influence the work of others in the sub-discipline. We also consider submissions from adjacent research fields where the central advance of the study is of interest to physicists, for example material sciences, physical chemistry and technologies.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


