An analytical method for broadband acoustic analysis of 2D cavities containing or bounded by porous materials
IF 7.1
1区 工程技术
Q1 ENGINEERING, MECHANICAL
International Journal of Mechanical Sciences
Pub Date : 2024-09-18
DOI:10.1016/j.ijmecsci.2024.109717
引用次数: 0
Abstract
Real-life vibro-acoustic systems often involve porous treatments, resulting in complex-valued and frequency-dependent models that are challenging to solve. Traditional prediction techniques like the finite element (FE) method requires huge computational cost, especially in the mid to high frequency ranges. This paper develops a novel spectral dynamic stiffness (SDS) formulation, using very few number of degrees of freedom but describing the broadband acoustic behaviour of acoustic cavities with porous materials highly accurately. The method employs frequency-dependent shape function that satisfies exactly the (damped) Helmholtz equation to describe the (equivalent) acoustic pressure field, and also features an innovative approach to use the fast-convergent Modified Fourier series to describe any arbitrary acoustic BCs. Finally, the SDS matrices for cavities containing or bounded by porous materials are formulated in an analytical manner. It is demonstrated that the method exhibits a much higher computational efficiency over the FE package COMSOL, at least 6 times faster than the COMSOL with a similar level of accuracy, and benchmark solutions are provided. This promising method can provide a powerful tool for systems with porous materials that have frequency-dependent characteristics, paving the way for efficient and accurate acoustic analysis in complex engineering applications.
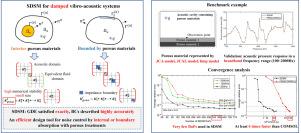
对含有多孔材料或以多孔材料为边界的二维空腔进行宽带声学分析的分析方法
现实生活中的振动声学系统往往涉及多孔处理,从而产生复杂的数值和频率相关模型,这对求解具有挑战性。传统的预测技术(如有限元(FE)方法)需要巨大的计算成本,尤其是在中高频率范围内。本文开发了一种新颖的频谱动态刚度(SDS)公式,使用很少的自由度,但能高度精确地描述多孔材料声腔的宽带声学行为。该方法采用了与频率相关的形状函数,完全满足(阻尼)亥姆霍兹方程来描述(等效)声压场,同时还采用了一种创新方法,使用快速收敛修正傅里叶级数来描述任意声波 BC。最后,以分析的方式制定了包含多孔材料或以多孔材料为边界的空腔的 SDS 矩阵。结果表明,与 FE 软件包 COMSOL 相比,该方法具有更高的计算效率,在精度相近的情况下至少比 COMSOL 快 6 倍,并提供了基准解。对于具有频率相关特性的多孔材料系统,这种前景广阔的方法可以提供一个强大的工具,为复杂工程应用中高效、准确的声学分析铺平道路。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

International Journal of Mechanical Sciences
工程技术-工程:机械
CiteScore
12.80
自引率
17.80%
发文量
769
审稿时长
19 days
期刊介绍:
The International Journal of Mechanical Sciences (IJMS) serves as a global platform for the publication and dissemination of original research that contributes to a deeper scientific understanding of the fundamental disciplines within mechanical, civil, and material engineering.
The primary focus of IJMS is to showcase innovative and ground-breaking work that utilizes analytical and computational modeling techniques, such as Finite Element Method (FEM), Boundary Element Method (BEM), and mesh-free methods, among others. These modeling methods are applied to diverse fields including rigid-body mechanics (e.g., dynamics, vibration, stability), structural mechanics, metal forming, advanced materials (e.g., metals, composites, cellular, smart) behavior and applications, impact mechanics, strain localization, and other nonlinear effects (e.g., large deflections, plasticity, fracture).
Additionally, IJMS covers the realms of fluid mechanics (both external and internal flows), tribology, thermodynamics, and materials processing. These subjects collectively form the core of the journal's content.
In summary, IJMS provides a prestigious platform for researchers to present their original contributions, shedding light on analytical and computational modeling methods in various areas of mechanical engineering, as well as exploring the behavior and application of advanced materials, fluid mechanics, thermodynamics, and materials processing.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


