Modeling of equivalent strain in 2D cross-sections of open die forged components using neural networks

Abstract

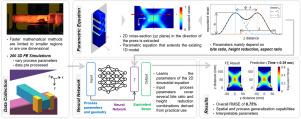
Open die forging is one of the oldest manufacturing methods known to remove defects in the ingot resulting from the casting process. The improved properties of the final component are highly dependent on the strain distribution. Although sinusoidal equations and empirical formulations have been already used to estimate the strain, they have been applied only to the core of the workpiece. In this work, a novel approach is presented to model the equivalent strain distribution in 2D cross-sections, in the direction of the press, of open die forged components using neural networks. The proposed method efficiently combines a parametric sinusoidal function with a neural network to learn the complex relationships between the process parameters and the resulting local strain. The neural network is trained on a dataset of finite element (FE) simulations of rectangular geometries that cover a wide range of aspect ratios, bite ratios, and height reductions. The presented methodology with near real-time prediction capabilities shows good agreement with FE results. Moreover, the parametric function captures the characteristic pattern of the strain distribution and reveals certain physical relationships affecting the deformation of the material. These patterns are later examined by analyzing the parameters identified in the parametric sinusoidal function.