Discrete differential geometry-based model for nonlinear analysis of axisymmetric shells

Abstract

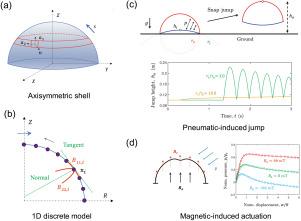
In this paper, we propose a novel one-dimensional (1D) discrete differential geometry (DDG)-based numerical method for geometrically nonlinear mechanics analysis (e.g., buckling and snapping) of axisymmetric shell structures. Our numerical model leverages differential geometry principles to accurately capture the complex nonlinear deformation patterns exhibited by axisymmetric shells. By discretizing the axisymmetric shell into interconnected 1D elements along the meridional direction, the in-plane stretching and out-of-bending potentials are formulated based on the geometric principles of 1D nodes and edges under the Kirchhoff–Love hypothesis, and elastic force vector and associated Hessian matrix required by equations of motion are later derived based on symbolic calculation. Through extensive validation with available theoretical solutions and finite element method (FEM) simulations in literature, our model demonstrates high accuracy in predicting the nonlinear behavior of axisymmetric shells. Importantly, compared to the classical theoretical model and three-dimensional (3D) FEM simulation, our model is highly computationally efficient, making it suitable for large-scale real-time simulations of nonlinear problems of shell structures such as instability and snap-through phenomena. Moreover, our framework can easily incorporate complex loading conditions, e.g., boundary nonlinear contact and multi-physics actuation, which play an essential role in the use of engineering applications, such as soft robots and flexible devices. This study demonstrates that the simplicity and effectiveness of the 1D discrete differential geometry-based approach render it a powerful tool for engineers and researchers interested in nonlinear mechanics analysis of axisymmetric shells, with potential applications in various engineering fields.