Active particle motion in Poiseuille flow through rectangular channels
IF 2.4
3区 物理与天体物理
Q1 Mathematics
引用次数: 0
Abstract
We investigate the dynamics of a pointlike active particle suspended in fluid flow through a straight channel. For this particle-fluid system, we derive a constant of motion for a general unidirectional fluid flow and apply it to an approximation of Poiseuille flow through channels with rectangular cross- sections. We obtain a nonlinear conservative dynamical system with one constant of motion and a dimensionless parameter describing the ratio of maximum flow speed to intrinsic active particle speed. Applied to square channels, we observe a diverse set of active particle trajectories with variations in system parameters and initial conditions which we classify into different types of swinging, trapping, tumbling, and wandering motion. Regular (periodic and quasiperiodic) motion as well as chaotic active particle motion are observed for these trajectories and quantified using largest Lyapunov exponents. We explore the transition to chaotic motion using Poincaré maps and show “sticky” chaotic tumbling trajectories that have long transients near a periodic state. We briefly illustrate how these results extend to rectangular cross-sections with a width-to-height ratio larger than one. Outcomes of this paper may have implications for dynamics of natural and artificial microswimmers in experimental microfluidic channels that typically have rectangular cross sections.
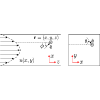
流经矩形水道的波瓦耶水流中的主动颗粒运动
我们研究了悬浮于流体中的点状活性粒子在直槽中的动力学。对于这个粒子-流体系统,我们推导出了一般单向流体流动的运动常数,并将其应用于矩形截面通道中的近似普瓦休耶(Poiseuille)流。我们得到了一个 4D 非线性保守动力系统,该系统具有一个运动常数和一个无量纲参数,该参数描述了最大流速与固有活动粒子速度之比。在方形水道中,我们观察到了多种多样的活动粒子轨迹,这些轨迹随着系统参数和初始条件的变化而变化,我们将其分为摆动、捕获、翻滚和徘徊等不同类型的运动。我们观察到了这些轨迹的规则(周期和准周期)运动以及混乱的主动粒子运动,并使用最大的 Lyapunov 指数对其进行量化。我们利用波恩卡雷图探索了向混沌运动的过渡,并展示了在周期状态附近具有较长瞬态的 "粘性 "混沌翻滚轨迹。我们简要说明了这些结果如何扩展到宽高比大于 1 的矩形截面。本文的结果可能会对通常具有矩形横截面的实验微流体通道中的天然和人工微泳者的动力学产生影响。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Physical review. E
物理-物理:流体与等离子体
CiteScore
4.60
自引率
16.70%
发文量
0
审稿时长
3.3 months
期刊介绍:
Physical Review E (PRE), broad and interdisciplinary in scope, focuses on collective phenomena of many-body systems, with statistical physics and nonlinear dynamics as the central themes of the journal. Physical Review E publishes recent developments in biological and soft matter physics including granular materials, colloids, complex fluids, liquid crystals, and polymers. The journal covers fluid dynamics and plasma physics and includes sections on computational and interdisciplinary physics, for example, complex networks.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


