A Novel Operational Matrix Method for Solving the Fractional Delay Integro-Differential Equations with a Weakly Singular Kernel
IF 1.4
4区 综合性期刊
Q2 MULTIDISCIPLINARY SCIENCES
Iranian Journal of Science and Technology, Transactions A: Science
Pub Date : 2024-07-30
DOI:10.1007/s40995-024-01682-0
引用次数: 0
Abstract
In this work, we introduce a feasible and efficient method for solving weakly singular fractional pantograph delay integro-differential equations. To implement the proposed method, we get the operational matrices based on the shifted fractional-order fifth-kind Chebyshev polynomials. These matrices, together with the collocation method, are applied to convert the main equation to a system of algebraic equations. We consider the existence and uniqueness of solutions and then give an upper error bound for this method. At last, several numerical tests are carried out to demonstrate the usefulness and capability of the suggested algorithm.
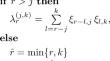
用弱奇异内核求解分式延迟积分微分方程的新型运算矩阵法
在这项工作中,我们介绍了一种解决弱奇异分式受电弓延迟积分微分方程的可行而高效的方法。为了实现所提出的方法,我们根据移位分式五阶切比雪夫多项式得到了运算矩阵。应用这些矩阵和配位法,可将主方程转换为代数方程系。我们考虑了解的存在性和唯一性,然后给出了这种方法的误差上限。最后,我们进行了几项数值测试,以证明所建议算法的实用性和能力。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Iranian Journal of Science and Technology, Transactions A: Science
MULTIDISCIPLINARY SCIENCES-
CiteScore
4.00
自引率
5.90%
发文量
122
审稿时长
>12 weeks
期刊介绍:
The aim of this journal is to foster the growth of scientific research among Iranian scientists and to provide a medium which brings the fruits of their research to the attention of the world’s scientific community. The journal publishes original research findings – which may be theoretical, experimental or both - reviews, techniques, and comments spanning all subjects in the field of basic sciences, including Physics, Chemistry, Mathematics, Statistics, Biology and Earth Sciences
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


