Some gradient theories in linear visco-elastodynamics towards dispersion and attenuation of waves in relation to large-strain models

IF 2.3 3区工程技术 Q2 MECHANICS

Acta Mechanica Pub Date : 2024-06-08 DOI:10.1007/s00707-024-03959-2

Tomáš Roubíček

引用次数: 0

Abstract

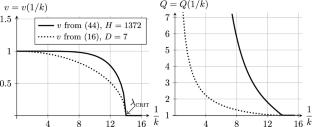
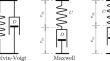
Various spatial-gradient extensions of standard viscoelastic rheologies of the Kelvin–Voigt, Maxwell’s, and Jeffreys’ types are analysed in linear one-dimensional situations as far as the propagation of waves and their dispersion and attenuation. These gradient extensions are then presented in the large-strain nonlinear variants where they are sometimes used rather for purely analytical reasons either in the Lagrangian or the Eulerian formulations without realizing this wave propagation context. The interconnection between these two modelling aspects is thus revealed in particular selected cases.

Abstract Image

查看原文本刊更多论文

线性粘弹性流体力学中的一些梯度理论：与大应变模型有关的波的分散和衰减

本文分析了开尔文-伏依格特、麦克斯韦和杰弗里斯类型的标准粘弹性流变学在一维线性情况下的各种空间梯度扩展，包括波的传播、波的扩散和衰减。然后，在大应变非线性变体中介绍了这些梯度扩展，在拉格朗日或欧拉公式中，有时出于纯粹的分析原因而使用这些梯度扩展，而没有意识到波的传播背景。因此，这两个建模方面的相互联系在特定的选定案例中得到了揭示。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Acta Mechanica 物理-力学

CiteScore

4.30

自引率

14.80%

发文量

292

审稿时长

6.9 months

期刊介绍： Since 1965, the international journal Acta Mechanica has been among the leading journals in the field of theoretical and applied mechanics. In addition to the classical fields such as elasticity, plasticity, vibrations, rigid body dynamics, hydrodynamics, and gasdynamics, it also gives special attention to recently developed areas such as non-Newtonian fluid dynamics, micro/nano mechanics, smart materials and structures, and issues at the interface of mechanics and materials. The journal further publishes papers in such related fields as rheology, thermodynamics, and electromagnetic interactions with fluids and solids. In addition, articles in applied mathematics dealing with significant mechanics problems are also welcome.