A weak theory of building blocks

IF 0.4 4区数学 Q4 LOGIC

Mathematical Logic Quarterly Pub Date : 2024-05-27 DOI:10.1002/malq.202300015

Juvenal Murwanashyaka

引用次数: 0

Abstract

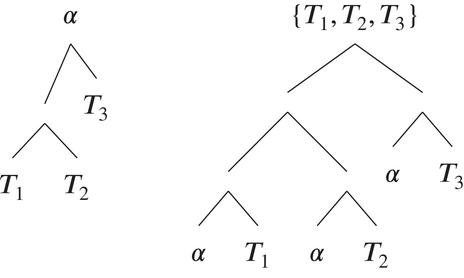
We apply the mereological concept of parthood to the coding of finite sequences. We propose a first-order theory in which coding finite sequences is intuitive and transparent. We compare this theory with Robinson arithmetic, adjunctive set theory and weak theories of finite strings and finite trees using interpretability.

Abstract Image

查看原文本刊更多论文

积木的弱理论

我们将parthood这一纯理论概念应用于有限序列的编码。我们提出了一种一阶理论，在这种理论中，有限序列编码是直观和透明的。我们利用可解释性将这一理论与罗宾逊算术、附属集合论以及有限字符串和有限树的弱理论进行了比较。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Mathematical Logic Quarterly 数学-数学

CiteScore

0.60

自引率

0.00%

发文量

审稿时长

>12 weeks

期刊介绍： Mathematical Logic Quarterly publishes original contributions on mathematical logic and foundations of mathematics and related areas, such as general logic, model theory, recursion theory, set theory, proof theory and constructive mathematics, algebraic logic, nonstandard models, and logical aspects of theoretical computer science.