Simple projection method: a novel algorithm for estimation of characteristic element length in finite element simulations of composites

Abstract

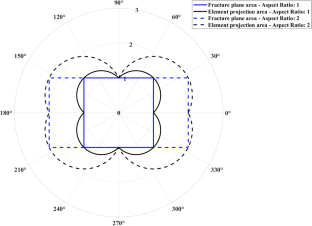
Mesh size dependency caused by strain localization is an ongoing problem in numerical simulations using the finite element method. In order to solve this problem, the concept of including the characteristic element length for regularization is used in the literature. The estimation of the characteristic element length is not a straightforward task since normally the characteristic element length differs from one element to another in the simulation and depends not only on element geometry but also on fracture plane orientation and material orientation. In this paper, an innovative method is proposed to estimate the characteristic element length which works on the orthogonal projection of elements on the fracture plane. The method is implemented in Abaqus/Explicit finite element solver and is verified using simple and more complex load cases such as tensile specimens, open hole specimens, and low-velocity impact. A good correlation between the numerical and experimental results in all of the studied cases was achieved and the proposed method proved to be effective in reducing mesh sensitivity. The use of the volumetric method from the literature for the simulation of open-hole tensile specimens led to more than 25% increase in the estimation of specimen strength while similar values of strength for different element aspect ratios were achieved with the proposed method.