Linear Instability of Symmetric Logarithmic Spiral Vortex Sheets

IF 1.2 3区数学 Q2 MATHEMATICS, APPLIED

Journal of Mathematical Fluid Mechanics Pub Date : 2024-02-23 DOI:10.1007/s00021-023-00847-y

Tomasz Cieślak, Piotr Kokocki, Wojciech S. Ożański

引用次数: 0

Abstract

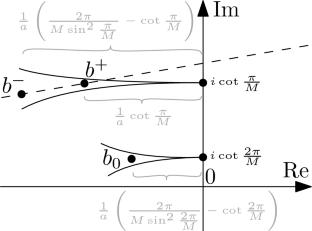
We consider Alexander spirals with \(M\ge 3\) branches, that is symmetric logarithmic spiral vortex sheets. We show that such vortex sheets are linearly unstable in the \(L^\infty \) (Kelvin–Helmholtz) sense, as solutions to the Birkhoff–Rott equation. To this end we consider Fourier modes in a logarithmic variable to identify unstable solutions with polynomial growth in time.

Abstract Image

查看原文本刊更多论文

对称对数螺旋涡旋片的线性不稳定性

Abstract We consider Alexander spirals with \(M\ge 3\) branches, that is symmetric logarithmic spiral vortex sheets.我们证明，作为伯克霍夫-罗特方程的解，这种涡旋片在（L^\infty \）（Kelvin-Helmholtz）意义上是线性不稳定的。为此，我们考虑了对数变量中的傅立叶模式，以确定在时间上具有多项式增长的不稳定解。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

求助全文

约1分钟内获得全文求助全文

来源期刊

Journal of Mathematical Fluid Mechanics 物理-力学

CiteScore

2.00

自引率

15.40%

发文量

审稿时长

>12 weeks

期刊介绍： The Journal of Mathematical Fluid Mechanics (JMFM)is a forum for the publication of high-quality peer-reviewed papers on the mathematical theory of fluid mechanics, with special regards to the Navier-Stokes equations. As an important part of that, the journal encourages papers dealing with mathematical aspects of computational theory, as well as with applications in science and engineering. The journal also publishes in related areas of mathematics that have a direct bearing on the mathematical theory of fluid mechanics. All papers will be characterized by originality and mathematical rigor. For a paper to be accepted, it is not enough that it contains original results. In fact, results should be highly relevant to the mathematical theory of fluid mechanics, and meet a wide readership.