Planar Rosa: a family of quasiperiodic substitution discrete plane tilings with 2n-fold rotational symmetry
IF 1.6
4区 计算机科学
Q3 COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCE
引用次数: 0
Abstract
We present Planar Rosa, a family of rhombus tilings with a 2n-fold rotational symmetry that are generated by a primitive substitution and that are also discrete plane tilings, meaning that they are obtained as a projection of a higher dimensional discrete plane. The discrete plane condition is a relaxed version of the cut-and-project condition. We also prove that the Sub Rosa substitution tilings with 2n-fold rotational symmetry defined by Kari and Rissanen do not satisfy even the weaker discrete plane condition. We prove these results for all even \(n\geqslant 4\). This completes our previously published results for odd values of n.
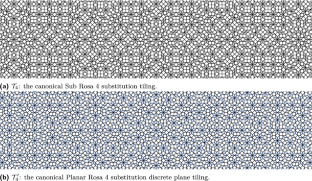
平面Rosa:一类具有2n倍旋转对称的准周期替代离散平面瓷砖
我们提出了平面罗莎,一个具有2n倍旋转对称的菱形瓷砖家族,它是由原始替换产生的,也是离散平面瓷砖,这意味着它们是作为高维离散平面的投影获得的。离散平面条件是切割-投影条件的松弛版本。我们还证明了由Kari和Rissanen定义的具有2n重旋转对称的Sub - Rosa替换瓷砖甚至不满足较弱的离散平面条件。我们对所有甚至\(n\geqslant 4\)证明了这些结果。这完成了我们之前发表的关于奇数n的结果。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Natural Computing
Computer Science-Computer Science Applications
CiteScore
4.40
自引率
4.80%
发文量
49
审稿时长
3 months
期刊介绍:
The journal is soliciting papers on all aspects of natural computing. Because of the interdisciplinary character of the journal a special effort will be made to solicit survey, review, and tutorial papers which would make research trends in a given subarea more accessible to the broad audience of the journal.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


