PRESCRIBED VIRTUAL HOMOLOGICAL TORSION OF 3-MANIFOLDS
IF 1.1
2区 数学
Q1 MATHEMATICS
Journal of the Institute of Mathematics of Jussieu
Pub Date : 2022-06-08
DOI:10.1017/s1474748022000329
引用次数: 1
Abstract
Let M be an irreducible 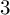 $3$-manifold M with empty or toroidal boundary which has at least one hyperbolic piece in its geometric decomposition, and let A be a finite abelian group. Generalizing work of Sun [20] and of Friedl–Herrmann [7], we prove that there exists a finite cover
$3$-manifold M with empty or toroidal boundary which has at least one hyperbolic piece in its geometric decomposition, and let A be a finite abelian group. Generalizing work of Sun [20] and of Friedl–Herrmann [7], we prove that there exists a finite cover 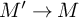 $M' \to M$ so that A is a direct factor in
$M' \to M$ so that A is a direct factor in 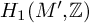 $H_1(M',{\mathbb Z})$.
$H_1(M',{\mathbb Z})$.
3流形的规定虚同调扭转
设M为不可约$3$流形M,其边界为空或环面,且其几何分解中至少有一个双曲块,设A为有限阿贝尔群。推广Sun[20]和Friedl-Herrmann[7]的工作,证明了存在一个有限覆盖$M' \到$M $,使得a是$H_1(M',{\mathbb Z})$的直接因子。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊
CiteScore
2.40
自引率
0.00%
发文量
54
审稿时长
>12 weeks
期刊介绍:
The Journal of the Institute of Mathematics of Jussieu publishes original research papers in any branch of pure mathematics; papers in logic and applied mathematics will also be considered, particularly when they have direct connections with pure mathematics. Its policy is to feature a wide variety of research areas and it welcomes the submission of papers from all parts of the world. Selection for publication is on the basis of reports from specialist referees commissioned by the Editors.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


