Viscoelastic behavior of composite materials with multi-coated ellipsoidal reinforcements and imperfect interfaces modeled by an equivalent inclusion

Abstract

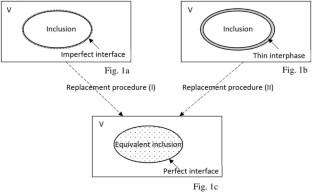
In this work, the effective behavior of viscoelastic composites with ellipsoidal reinforcements and imperfect interface or degraded interphase is investigated through the inclusion replacement concept. The concentration equations have been reformulated as to define the equivalent inclusion’s behavior with imperfect interface or thin coating allowing to evaluate the effective behavior through different homogenization schemes. The correlation between interface and interphase descriptions is formulated in the context of anisotropic behavior of the inclusion and the matrix and for ellipsoidal inclusion shape. In the case of isotropic elasticity, the exact analytical solutions agree with the literature references for spherical and cylindrical inclusion morphologies and linear spring interface model. The replacement procedure was extended to viscoelastic behavior of the components with imperfect interface and/or interphase. Alternative descriptions of the interface behavior are proposed through Maxwell and Kelvin–Voigt models. The combined influence of shape of inclusions and interface parameters is analyzed on the effective relaxation modulus.