On von Neumann regularity of cellular automata
IF 1.6
4区 计算机科学
Q3 COMPUTER SCIENCE, ARTIFICIAL INTELLIGENCE
引用次数: 0
Abstract
Abstract We show that a cellular automaton on a one-dimensional two-sided mixing subshift of finite type is a von Neumann regular element in the semigroup of cellular automata if and only if it is split epic onto its image in the category of sofic shifts and block maps. It follows from previous joint work of the author and Törmä that von Neumann regularity is a decidable condition, and we decide it for all elementary CA, obtaining the optimal radii for weak generalized inverses. Two sufficient conditions for non-regularity are having a proper sofic image or having a point in the image with no preimage of the same period. We show that the non-regular ECA 9 and 28 cannot be proven non-regular using these methods. We also show that a random cellular automaton is non-regular with high probability.
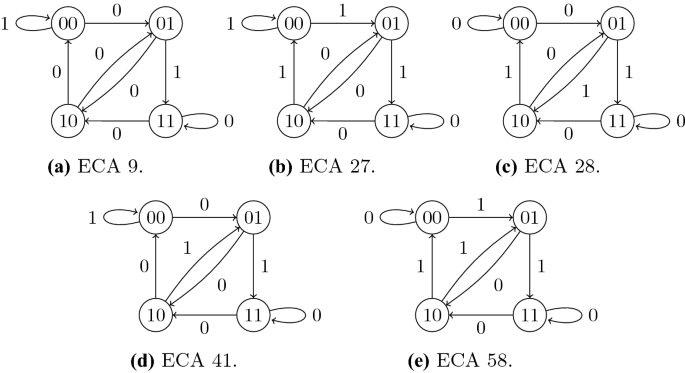
元胞自动机的von Neumann正则性
摘要本文证明了一维有限型双向混合子位移上的元胞自动机是元胞自动机半群中的von Neumann正则元素,当且仅当它在微位移和块映射的范畴内被分割成它的像。从作者和Törmä前人的共同工作中得出von Neumann正则性是一个可判定的条件,并对所有初等CA都判定了它,得到了弱广义逆的最优半径。非正则性的两个充分条件是有一个正确的图像或图像中有一个点没有同时期的预像。我们表明,使用这些方法不能证明非规则ECA 9和28是非规则的。我们还证明了随机元胞自动机具有高概率的非规则性。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Natural Computing
Computer Science-Computer Science Applications
CiteScore
4.40
自引率
4.80%
发文量
49
审稿时长
3 months
期刊介绍:
The journal is soliciting papers on all aspects of natural computing. Because of the interdisciplinary character of the journal a special effort will be made to solicit survey, review, and tutorial papers which would make research trends in a given subarea more accessible to the broad audience of the journal.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


