Hydrodynamic regime and cold plasmas hit by short laser pulses
IF 1.1
4区 数学
Q1 MATHEMATICS
引用次数: 0
Abstract
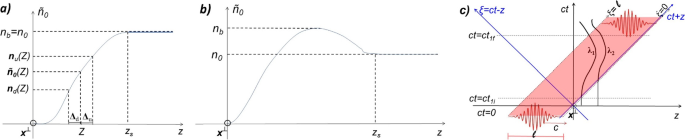
We briefly report and elaborate on some conditions allowing a hydrodynamic description of the impact of a very short and arbitrarily intense laser pulse onto a cold plasma, as well as the localization of the first wave-breaking due to the plasma inhomogeneity. We use a recently developed fully relativistic plane model whereby we reduce the system of the Lorentz-Maxwell and continuity PDEs into a 1-parameter family of decoupled systems of non-autonomous Hamilton equations in dimension 1, with the light-like coordinate $\xi=ct\!-\!z$ replacing time $t$ as an independent variable. Apriori estimates on the Jacobian $\hat J$ of the change from Lagrangian to Eulerian coordinates in terms of the input data (initial density and pulse profile) are obtained applying Liapunov direct method to an associated family of pairs of ODEs; wave-breaking is pinpointed by the inequality $\hat J\le 0$. These results may help in drastically simplifying the study of extreme acceleration mechanisms of electrons, which have very important applications.
短激光脉冲冲击下的流体动力学和冷等离子体
我们简要地报告并详细说明了一些条件,这些条件允许对极短且任意强度的激光脉冲对冷等离子体的影响进行流体动力学描述,以及由于等离子体不均匀性而导致的第一次破波的定位。我们使用最近开发的完全相对论平面模型,在该模型中,我们将洛伦兹-麦克斯韦方程组和连续性偏微分方程简化为1维非自治汉密尔顿方程的1参数解耦系统族,用类光坐标$\xi=ct\!-\!z$代替时间$t$作为自变量。应用Liapunov直接法对相关的ode对族进行了先验估计,得到了输入数据(初始密度和脉冲轮廓)从拉格朗日坐标到欧拉坐标变化的雅可比矩阵$\hat J$的先验估计;破浪是由不等式$\hat J\le 0$确定的。这些结果可能有助于大大简化电子极端加速机制的研究,这具有非常重要的应用。
本文章由计算机程序翻译,如有差异,请以英文原文为准。
求助全文
约1分钟内获得全文
求助全文
来源期刊

Ricerche di Matematica
Mathematics-Applied Mathematics
CiteScore
3.00
自引率
8.30%
发文量
61
期刊介绍:
“Ricerche di Matematica” publishes high-quality research articles in any field of pure and applied mathematics. Articles must be original and written in English. Details about article submission can be found online.
 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


