Spectral and Energy–Lyapunov stability of streamwise Couette–Poiseuille and spanwise Poiseuille base flows

Abstract

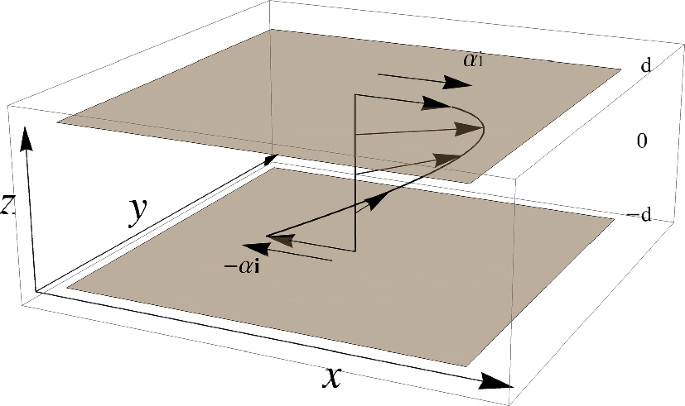
Abstract When a fluid fills an infinite layer between two rigid plates in relative motion, and it is simultaneously subject to a gradient of pressure not parallel to the motion, the base flow is a combination of Couette–Poiseuille in the direction along the boundaries’ relative motion, but it also possess a Poiseuille component in the transverse direction. For this reason the linearised equations include all variables x , y , z , and not only explicitly two variables x , z as it typically happens in the literature. For convenience, we indicate as streamwise the direction of the relative motions of the plates, and spanwise the orthogonal direction. We use Chebyshev collocation method to investigate the monotonic behaviour of the energy along perturbations of general streamwise Couette–Poiseuille plus spanwise Poiseuille base flow, thus obtaining energy-critical Reynolds numbers depending on two parameters. We finally compute the spectrum of the linearisation at such base flows, and hence determine spectrum-critical Reynolds numbers depending on the two parameters. The choice of convex combinations of Couette and Poiseuille flows along the streamwise direction, and spanwise Poiseuille flow, affects the value of the energy-critical Reynolds and wave numbers in interesting ways. Also the spectrum-critical Reynolds and wave numbers depend on the type of base flow in peculiar ways. These dependencies are not described in the literature.