时间信号和过去时间的时间因果和时间递归尺度-协变尺度-空间表示。
摘要
本文概述了对时间信号进行时间平滑的理论,如下所示:(i)较粗时间尺度上的时间平滑信号保证构成任何较细时间尺度(包括原始信号)上相应时间平滑信号的简化;(ii)时间平滑过程既是时间因果的,也是时间递归的;从某种意义上说,它不需要访问未来的信息,并且可以在没有其他过去的时间记忆缓冲的情况下执行,而不是结果平滑的时间尺度空间表示本身。对于符合这一性质的线性和移不变时间平滑算子类的参数设置的特定子集,展示了如何额外获得时间尺度协方差,从而保证如果时间输入信号被统一的时间尺度因子重新缩放,然后,由此产生的重新缩放的时间信号的时间尺度空间表示将构成原始输入信号的时间尺度空间表示的重新缩放,并辅之以沿着时间尺度维度的移位。当正则高斯核由于其对未来信息的非因果访问而无法使用时,遵循这一属性的时间-因果极限核构成了一个规范的时间核,用于处理实时场景中的时间信号,并且我们不能额外要求时间平滑过程包含时间平滑过程本身所包含的信息之外的过去的补充记忆。这种方式也可以作为过去的多尺度时间记忆。我们描述了时间因果极限核如何与以前使用的时间模型相关,例如Koenderink的尺度时间核和前高斯核。我们还概述了时间因果极限核如何用于时空和光谱时间接受场模型中的时间处理建模,以及它如何更普遍地具有以纯粹的时间因果和时间递归方式建模神经时间响应函数的高潜力,这也可以以理论上有充分根据的方式处理多个时间尺度的现象。我们详细介绍了该理论如何有效地实现离散数据,根据一组递归滤波器耦合级联。因此,该理论一般适用于:(i)在多个时间尺度上对连续时间现象进行建模,(ii)对实时测量的时间信号进行数字处理。最后,我们陈述了通过数学模型对生物、感知、神经和记忆过程中的时间现象建模的理论含义,以及关于时间哲学和感知代理的含义。具体来说,我们建议对于a型时间理论,以及对于感知主体,时间接受野的非无穷小内部时间尺度的概念必须包含在当前的表征中,其中这种时间因果接受野的固有非零时间延迟意味着需要将来自实际时间延迟的预测纳入感知层次的各层中。为了使感知现在的表征能够构成一个具有更接近实际现在的时序属性的环境表征。
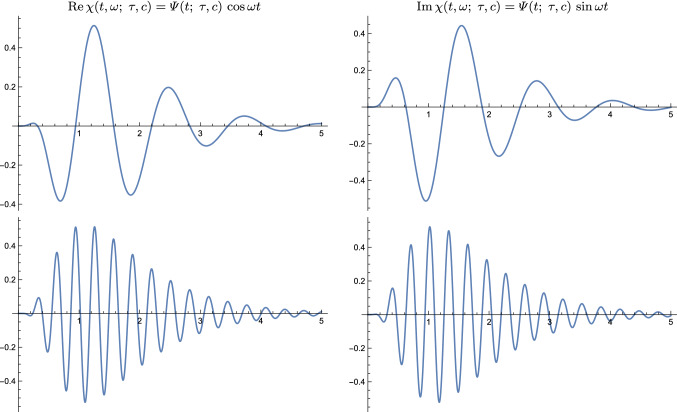

This article presents an overview of a theory for performing temporal smoothing on temporal signals in such a way that: (i) temporally smoothed signals at coarser temporal scales are guaranteed to constitute simplifications of corresponding temporally smoothed signals at any finer temporal scale (including the original signal) and (ii) the temporal smoothing process is both time-causal and time-recursive, in the sense that it does not require access to future information and can be performed with no other temporal memory buffer of the past than the resulting smoothed temporal scale-space representations themselves. For specific subsets of parameter settings for the classes of linear and shift-invariant temporal smoothing operators that obey this property, it is shown how temporal scale covariance can be additionally obtained, guaranteeing that if the temporal input signal is rescaled by a uniform temporal scaling factor, then also the resulting temporal scale-space representations of the rescaled temporal signal will constitute mere rescalings of the temporal scale-space representations of the original input signal, complemented by a shift along the temporal scale dimension. The resulting time-causal limit kernel that obeys this property constitutes a canonical temporal kernel for processing temporal signals in real-time scenarios when the regular Gaussian kernel cannot be used, because of its non-causal access to information from the future, and we cannot additionally require the temporal smoothing process to comprise a complementary memory of the past beyond the information contained in the temporal smoothing process itself, which in this way also serves as a multi-scale temporal memory of the past. We describe how the time-causal limit kernel relates to previously used temporal models, such as Koenderink's scale-time kernels and the ex-Gaussian kernel. We do also give an overview of how the time-causal limit kernel can be used for modelling the temporal processing in models for spatio-temporal and spectro-temporal receptive fields, and how it more generally has a high potential for modelling neural temporal response functions in a purely time-causal and time-recursive way, that can also handle phenomena at multiple temporal scales in a theoretically well-founded manner. We detail how this theory can be efficiently implemented for discrete data, in terms of a set of recursive filters coupled in cascade. Hence, the theory is generally applicable for both: (i) modelling continuous temporal phenomena over multiple temporal scales and (ii) digital processing of measured temporal signals in real time. We conclude by stating implications of the theory for modelling temporal phenomena in biological, perceptual, neural and memory processes by mathematical models, as well as implications regarding the philosophy of time and perceptual agents. Specifically, we propose that for A-type theories of time, as well as for perceptual agents, the notion of a non-infinitesimal inner temporal scale of the temporal receptive fields has to be included in representations of the present, where the inherent nonzero temporal delay of such time-causal receptive fields implies a need for incorporating predictions from the actual time-delayed present in the layers of a perceptual hierarchy, to make it possible for a representation of the perceptual present to constitute a representation of the environment with timing properties closer to the actual present.

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


