多出租车群体竞争模型

IF 0.58 Q3 Engineering

Journal of Applied and Industrial Mathematics Pub Date : 2023-11-04 DOI:10.1134/S1990478923030043

A. V. Budyansky, V. G. Tsybulin

{"title":"多出租车群体竞争模型","authors":"A. V. Budyansky, V. G. Tsybulin","doi":"10.1134/S1990478923030043","DOIUrl":null,"url":null,"abstract":"<p> We study a mathematical model of competition between two populations described by a\nsystem of nonlinear differential reaction–diffusion–advection equations. The taxis is introduced to\nmodel the heterogeneity of the total resource and the nonuniform distribution of both species. We\nanalyze the role of the taxis in the area occupancy. The maps of migration parameters\ncorresponding to various variants of competitive exclusion and coexistence of species are\ncalculated. Using the theory of cosymmetry, we find parametric relations under which\nmultistability arises. In a computational experiment, population scenarios with a violation of\ncosymmetry were studied.\n</p>","PeriodicalId":607,"journal":{"name":"Journal of Applied and Industrial Mathematics","volume":null,"pages":null},"PeriodicalIF":0.5800,"publicationDate":"2023-11-04","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Modeling Competition between Populations with Multi-Taxis\",\"authors\":\"A. V. Budyansky, V. G. Tsybulin\",\"doi\":\"10.1134/S1990478923030043\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<p> We study a mathematical model of competition between two populations described by a\\nsystem of nonlinear differential reaction–diffusion–advection equations. The taxis is introduced to\\nmodel the heterogeneity of the total resource and the nonuniform distribution of both species. We\\nanalyze the role of the taxis in the area occupancy. The maps of migration parameters\\ncorresponding to various variants of competitive exclusion and coexistence of species are\\ncalculated. Using the theory of cosymmetry, we find parametric relations under which\\nmultistability arises. In a computational experiment, population scenarios with a violation of\\ncosymmetry were studied.\\n</p>\",\"PeriodicalId\":607,\"journal\":{\"name\":\"Journal of Applied and Industrial Mathematics\",\"volume\":null,\"pages\":null},\"PeriodicalIF\":0.5800,\"publicationDate\":\"2023-11-04\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Journal of Applied and Industrial Mathematics\",\"FirstCategoryId\":\"1085\",\"ListUrlMain\":\"https://link.springer.com/article/10.1134/S1990478923030043\",\"RegionNum\":0,\"RegionCategory\":null,\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q3\",\"JCRName\":\"Engineering\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Journal of Applied and Industrial Mathematics","FirstCategoryId":"1085","ListUrlMain":"https://link.springer.com/article/10.1134/S1990478923030043","RegionNum":0,"RegionCategory":null,"ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q3","JCRName":"Engineering","Score":null,"Total":0}

引用次数: 0

摘要

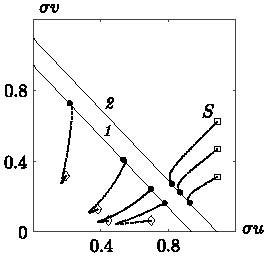
我们研究了一个由非线性微分反应-扩散-平流方程系统描述的两个种群之间竞争的数学模型。引入taxis来模拟总资源的异质性和两个物种的不均匀分布。分析出租车在区域占用中的作用。计算了响应物种竞争排斥和共存的各种变体的迁移参数图。利用共对称理论，我们找到了多稳定性产生的参数关系。在一个计算实验中，研究了违反对称性的人口场景。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

Modeling Competition between Populations with Multi-Taxis

查看原文本刊更多论文

Modeling Competition between Populations with Multi-Taxis

We study a mathematical model of competition between two populations described by a system of nonlinear differential reaction–diffusion–advection equations. The taxis is introduced to model the heterogeneity of the total resource and the nonuniform distribution of both species. We analyze the role of the taxis in the area occupancy. The maps of migration parameters corresponding to various variants of competitive exclusion and coexistence of species are calculated. Using the theory of cosymmetry, we find parametric relations under which multistability arises. In a computational experiment, population scenarios with a violation of cosymmetry were studied.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Journal of Applied and Industrial Mathematics Engineering-Industrial and Manufacturing Engineering

CiteScore

1.00

自引率

0.00%

发文量

期刊介绍： Journal of Applied and Industrial Mathematics is a journal that publishes original and review articles containing theoretical results and those of interest for applications in various branches of industry. The journal topics include the qualitative theory of differential equations in application to mechanics, physics, chemistry, biology, technical and natural processes; mathematical modeling in mechanics, physics, engineering, chemistry, biology, ecology, medicine, etc.; control theory; discrete optimization; discrete structures and extremum problems; combinatorics; control and reliability of discrete circuits; mathematical programming; mathematical models and methods for making optimal decisions; models of theory of scheduling, location and replacement of equipment; modeling the control processes; development and analysis of algorithms; synthesis and complexity of control systems; automata theory; graph theory; game theory and its applications; coding theory; scheduling theory; and theory of circuits.