范畴Galois理论中高覆盖的对称方法

IF 0.6 4区数学 Q3 MATHEMATICS

Applied Categorical Structures Pub Date : 2023-01-11 DOI:10.1007/s10485-022-09698-6

Fara Renaud, Tim Van der Linden

{"title":"范畴Galois理论中高覆盖的对称方法","authors":"Fara Renaud, Tim Van der Linden","doi":"10.1007/s10485-022-09698-6","DOIUrl":null,"url":null,"abstract":"<div><p>In the context of a tower of (strongly Birkhoff) Galois structures in the sense of categorical Galois theory, we show that the concept of a higher covering admits a characterisation which is at the same time <i>absolute</i> (with respect to the base level in the tower), rather than inductively defined relative to extensions of a lower order; and <i>symmetric</i>, rather than depending on a perspective in terms of arrows pointing in a certain chosen direction. This result applies to the Galois theory of quandles, for instance, where it helps us characterising the higher coverings in purely algebraic terms.\n</p></div>","PeriodicalId":7952,"journal":{"name":"Applied Categorical Structures","volume":"31 1","pages":""},"PeriodicalIF":0.6000,"publicationDate":"2023-01-11","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"https://link.springer.com/content/pdf/10.1007/s10485-022-09698-6.pdf","citationCount":"0","resultStr":"{\"title\":\"A symmetric approach to higher coverings in categorical Galois theory\",\"authors\":\"Fara Renaud, Tim Van der Linden\",\"doi\":\"10.1007/s10485-022-09698-6\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"<div><p>In the context of a tower of (strongly Birkhoff) Galois structures in the sense of categorical Galois theory, we show that the concept of a higher covering admits a characterisation which is at the same time <i>absolute</i> (with respect to the base level in the tower), rather than inductively defined relative to extensions of a lower order; and <i>symmetric</i>, rather than depending on a perspective in terms of arrows pointing in a certain chosen direction. This result applies to the Galois theory of quandles, for instance, where it helps us characterising the higher coverings in purely algebraic terms.\\n</p></div>\",\"PeriodicalId\":7952,\"journal\":{\"name\":\"Applied Categorical Structures\",\"volume\":\"31 1\",\"pages\":\"\"},\"PeriodicalIF\":0.6000,\"publicationDate\":\"2023-01-11\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"https://link.springer.com/content/pdf/10.1007/s10485-022-09698-6.pdf\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Applied Categorical Structures\",\"FirstCategoryId\":\"100\",\"ListUrlMain\":\"https://link.springer.com/article/10.1007/s10485-022-09698-6\",\"RegionNum\":4,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q3\",\"JCRName\":\"MATHEMATICS\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Applied Categorical Structures","FirstCategoryId":"100","ListUrlMain":"https://link.springer.com/article/10.1007/s10485-022-09698-6","RegionNum":4,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q3","JCRName":"MATHEMATICS","Score":null,"Total":0}

引用次数: 0

摘要

在范畴伽罗瓦理论意义上的(强Birkhoff)伽罗瓦结构塔的背景下，我们证明了更高覆盖的概念承认同时是绝对的特征(相对于塔中的基础水平)，而不是相对于低阶的扩展归纳定义;而且是对称的，而不是依赖于箭头指向某个特定方向的角度。这个结果适用于伽罗瓦的量子理论，例如，它帮助我们用纯代数术语来描述高覆盖。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

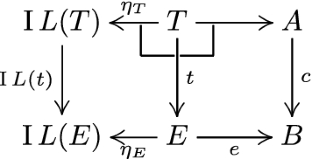
A symmetric approach to higher coverings in categorical Galois theory

查看原文本刊更多论文

A symmetric approach to higher coverings in categorical Galois theory

In the context of a tower of (strongly Birkhoff) Galois structures in the sense of categorical Galois theory, we show that the concept of a higher covering admits a characterisation which is at the same time absolute (with respect to the base level in the tower), rather than inductively defined relative to extensions of a lower order; and symmetric, rather than depending on a perspective in terms of arrows pointing in a certain chosen direction. This result applies to the Galois theory of quandles, for instance, where it helps us characterising the higher coverings in purely algebraic terms.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Applied Categorical Structures 数学-数学

CiteScore

1.30

自引率

16.70%

发文量

审稿时长

>12 weeks

期刊介绍： Applied Categorical Structures focuses on applications of results, techniques and ideas from category theory to mathematics, physics and computer science. These include the study of topological and algebraic categories, representation theory, algebraic geometry, homological and homotopical algebra, derived and triangulated categories, categorification of (geometric) invariants, categorical investigations in mathematical physics, higher category theory and applications, categorical investigations in functional analysis, in continuous order theory and in theoretical computer science. In addition, the journal also follows the development of emerging fields in which the application of categorical methods proves to be relevant. Applied Categorical Structures publishes both carefully refereed research papers and survey papers. It promotes communication and increases the dissemination of new results and ideas among mathematicians and computer scientists who use categorical methods in their research.