具有lsamvy跃变和媒体报道的随机SIR流行病模型。

IF 4.1 3区数学 Q1 Mathematics

Advances in Difference Equations Pub Date : 2020-01-01 Epub Date: 2020-02-12 DOI:10.1186/s13662-020-2521-6

Yingfen Liu, Yan Zhang, Qingyun Wang

{"title":"具有lsamvy跃变和媒体报道的随机SIR流行病模型。","authors":"Yingfen Liu, Yan Zhang, Qingyun Wang","doi":"10.1186/s13662-020-2521-6","DOIUrl":null,"url":null,"abstract":"A stochastic susceptible-infectious-recovered epidemic model with temporary immunity and media coverage is proposed. The effects of Lévy jumps on the dynamics of the model are considered. A unique global positive solution for the epidemic model is obtained. Sufficient conditions are derived to guarantee that the epidemic disease is extinct and persistent in the mean. The threshold behavior is discussed. Numerical simulations are given to verify our theoretical results.","PeriodicalId":53311,"journal":{"name":"Advances in Difference Equations","volume":"2020 1","pages":"70"},"PeriodicalIF":4.1000,"publicationDate":"2020-01-01","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"https://sci-hub-pdf.com/10.1186/s13662-020-2521-6","citationCount":"2","resultStr":"{\"title\":\"A stochastic SIR epidemic model with Lévy jump and media coverage.\",\"authors\":\"Yingfen Liu, Yan Zhang, Qingyun Wang\",\"doi\":\"10.1186/s13662-020-2521-6\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"A stochastic susceptible-infectious-recovered epidemic model with temporary immunity and media coverage is proposed. The effects of Lévy jumps on the dynamics of the model are considered. A unique global positive solution for the epidemic model is obtained. Sufficient conditions are derived to guarantee that the epidemic disease is extinct and persistent in the mean. The threshold behavior is discussed. Numerical simulations are given to verify our theoretical results.\",\"PeriodicalId\":53311,\"journal\":{\"name\":\"Advances in Difference Equations\",\"volume\":\"2020 1\",\"pages\":\"70\"},\"PeriodicalIF\":4.1000,\"publicationDate\":\"2020-01-01\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"https://sci-hub-pdf.com/10.1186/s13662-020-2521-6\",\"citationCount\":\"2\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Advances in Difference Equations\",\"FirstCategoryId\":\"100\",\"ListUrlMain\":\"https://doi.org/10.1186/s13662-020-2521-6\",\"RegionNum\":3,\"RegionCategory\":\"数学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"2020/2/12 0:00:00\",\"PubModel\":\"Epub\",\"JCR\":\"Q1\",\"JCRName\":\"Mathematics\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Advances in Difference Equations","FirstCategoryId":"100","ListUrlMain":"https://doi.org/10.1186/s13662-020-2521-6","RegionNum":3,"RegionCategory":"数学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"2020/2/12 0:00:00","PubModel":"Epub","JCR":"Q1","JCRName":"Mathematics","Score":null,"Total":0}

引用次数: 2

摘要

提出了一种具有暂时免疫和媒介覆盖的随机易感-感染-恢复流行病模型。考虑了lsamvy跃变对模型动力学的影响。得到了流行病模型的唯一全局正解。导出了保证传染病绝灭并在均值上持续存在的充分条件。讨论了阈值行为。通过数值模拟验证了理论结果。

本文章由计算机程序翻译，如有差异，请以英文原文为准。

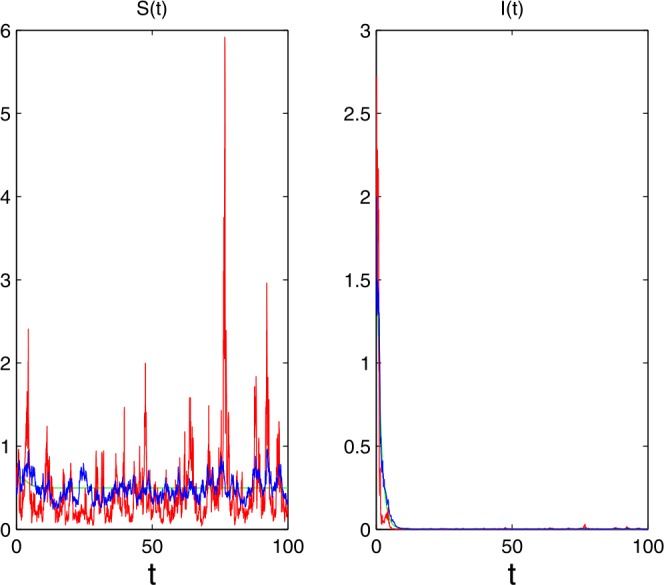
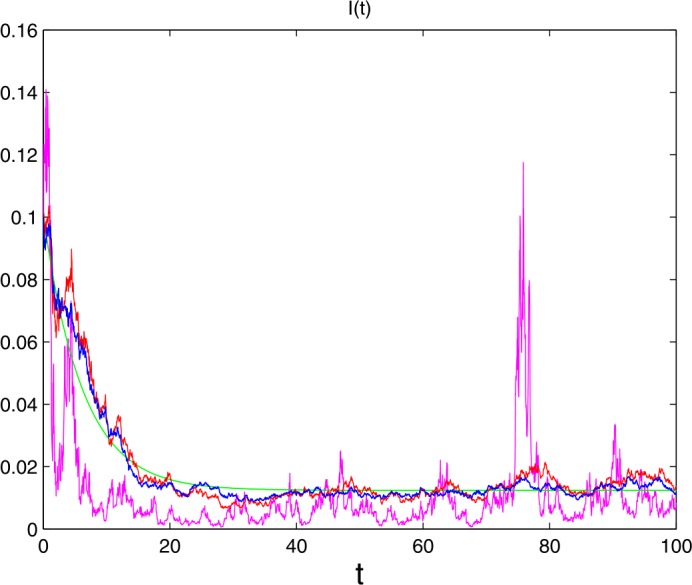
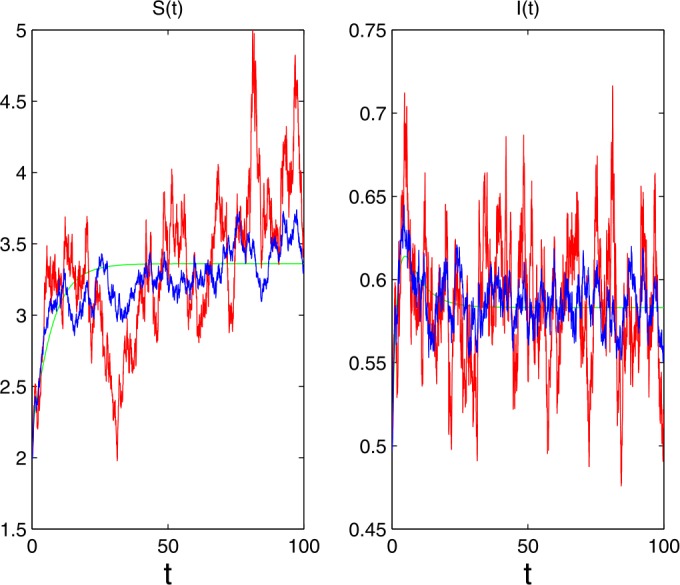
A stochastic SIR epidemic model with Lévy jump and media coverage.

查看原文本刊更多论文

A stochastic SIR epidemic model with Lévy jump and media coverage.

A stochastic susceptible-infectious-recovered epidemic model with temporary immunity and media coverage is proposed. The effects of Lévy jumps on the dynamics of the model are considered. A unique global positive solution for the epidemic model is obtained. Sufficient conditions are derived to guarantee that the epidemic disease is extinct and persistent in the mean. The threshold behavior is discussed. Numerical simulations are given to verify our theoretical results.

求助全文

通过发布文献求助，成功后即可免费获取论文全文。去求助

来源期刊

Advances in Difference Equations 数学-数学

自引率

0.00%

发文量

审稿时长

4-8 weeks

期刊介绍： The theory of difference equations, the methods used, and their wide applications have advanced beyond their adolescent stage to occupy a central position in applicable analysis. In fact, in the last 15 years, the proliferation of the subject has been witnessed by hundreds of research articles, several monographs, many international conferences, and numerous special sessions. The theory of differential and difference equations forms two extreme representations of real world problems. For example, a simple population model when represented as a differential equation shows the good behavior of solutions whereas the corresponding discrete analogue shows the chaotic behavior. The actual behavior of the population is somewhere in between. The aim of Advances in Difference Equations is to report mainly the new developments in the field of difference equations, and their applications in all fields. We will also consider research articles emphasizing the qualitative behavior of solutions of ordinary, partial, delay, fractional, abstract, stochastic, fuzzy, and set-valued differential equations. Advances in Difference Equations will accept high-quality articles containing original research results and survey articles of exceptional merit.