R. Merkle, M.F. Hoedl, A. Chesnokov, D. Gryaznov, E. Bucher, E.A. Kotomin, W. Sitte, J. Maier
求助PDF
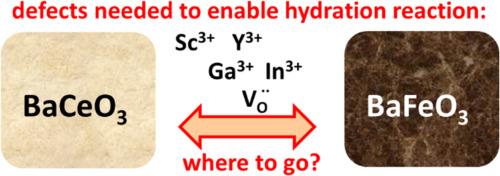
{"title":"Ba(Ce,Fe,Acc)O3-δ体系的电子结构、相形成及缺陷分布","authors":"R. Merkle, M.F. Hoedl, A. Chesnokov, D. Gryaznov, E. Bucher, E.A. Kotomin, W. Sitte, J. Maier","doi":"10.1016/j.actamat.2025.120739","DOIUrl":null,"url":null,"abstract":"Composites of two perovskites are one possibility to combine protonic and p-type electronic conductivity as required for oxygen electrodes in protonic ceramic electrochemical cells. The BaCeO<sub>3</sub>-BaFeO<sub>3</sub> system can be acceptor-doped to increase proton uptake and transport. However, preceding experiments [C. Berger et al., J. Mater. Chem. A 10 (2022) 2474; C. Nader et al., Solid State Ionics 406 (2024) 116474] indicated that the dopants are inhomogeneously distributed between the two phases, which is decisive for hydration ability and proton conductivity of such composites. Here, we use extended density functional theory calculations (DFT+U, Hubbard approach) for a comprehensive characterization of the BaCeO<sub>3</sub>-BaFeO<sub>3</sub> system including acceptors. Supercells of various compositions are calculated to derive chemical reaction energies, for example for the transfer of defects between the phases. Two key aspects related to the hydration ability of such materials are: (i) The development of the electronic structure with increasing Fe content in a (hypothetical) single-phase BaCe<sub>1-x</sub>Fe<sub>x</sub>O<sub>3</sub> perovskite. (ii) The distribution of acceptors (Ga<sup>3+</sup>, Sc<sup>3+</sup>, In<sup>3+</sup>, Y<sup>3+</sup>) and oxygen vacancies (<span><span style=\"\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">&#x2022;</mo><mo is=\"true\">&#x2022;</mo></mrow></msubsup></math>' role=\"presentation\" style=\"font-size: 90%; display: inline-block; position: relative;\" tabindex=\"0\"><svg aria-hidden=\"true\" focusable=\"false\" height=\"2.779ex\" role=\"img\" style=\"vertical-align: -1.043ex;\" viewbox=\"0 -747.2 1558.3 1196.3\" width=\"3.619ex\" xmlns:xlink=\"http://www.w3.org/1999/xlink\"><g fill=\"currentColor\" stroke=\"currentColor\" stroke-width=\"0\" transform=\"matrix(1 0 0 -1 0 0)\"><g is=\"true\"><g is=\"true\"><use xlink:href=\"#MJMAIN-56\"></use></g><g is=\"true\" transform=\"translate(750,306)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g><g is=\"true\" transform=\"translate(353,0)\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g></g><g is=\"true\" transform=\"translate(750,-335)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-4F\"></use></g></g></g></g></svg><span role=\"presentation\"><math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi is=\"true\" mathvariant=\"normal\">V</mi><mrow is=\"true\"><mi is=\"true\" mathvariant=\"normal\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></span></span><script type=\"math/mml\"><math><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></script></span>) between Ce- and Fe-rich phases. The segregation driving forces of acceptor dopant and <span><span style=\"\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">&#x2022;</mo><mo is=\"true\">&#x2022;</mo></mrow></msubsup></math>' role=\"presentation\" style=\"font-size: 90%; display: inline-block; position: relative;\" tabindex=\"0\"><svg aria-hidden=\"true\" focusable=\"false\" height=\"2.779ex\" role=\"img\" style=\"vertical-align: -1.043ex;\" viewbox=\"0 -747.2 1558.3 1196.3\" width=\"3.619ex\" xmlns:xlink=\"http://www.w3.org/1999/xlink\"><g fill=\"currentColor\" stroke=\"currentColor\" stroke-width=\"0\" transform=\"matrix(1 0 0 -1 0 0)\"><g is=\"true\"><g is=\"true\"><use xlink:href=\"#MJMAIN-56\"></use></g><g is=\"true\" transform=\"translate(750,306)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g><g is=\"true\" transform=\"translate(353,0)\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g></g><g is=\"true\" transform=\"translate(750,-335)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-4F\"></use></g></g></g></g></svg><span role=\"presentation\"><math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi is=\"true\" mathvariant=\"normal\">V</mi><mrow is=\"true\"><mi is=\"true\" mathvariant=\"normal\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></span></span><script type=\"math/mml\"><math><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></script></span> are calculated individually. <span><span style=\"\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">&#x2022;</mo><mo is=\"true\">&#x2022;</mo></mrow></msubsup></math>' role=\"presentation\" style=\"font-size: 90%; display: inline-block; position: relative;\" tabindex=\"0\"><svg aria-hidden=\"true\" focusable=\"false\" height=\"2.779ex\" role=\"img\" style=\"vertical-align: -1.043ex;\" viewbox=\"0 -747.2 1558.3 1196.3\" width=\"3.619ex\" xmlns:xlink=\"http://www.w3.org/1999/xlink\"><g fill=\"currentColor\" stroke=\"currentColor\" stroke-width=\"0\" transform=\"matrix(1 0 0 -1 0 0)\"><g is=\"true\"><g is=\"true\"><use xlink:href=\"#MJMAIN-56\"></use></g><g is=\"true\" transform=\"translate(750,306)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g><g is=\"true\" transform=\"translate(353,0)\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g></g><g is=\"true\" transform=\"translate(750,-335)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-4F\"></use></g></g></g></g></svg><span role=\"presentation\"><math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi is=\"true\" mathvariant=\"normal\">V</mi><mrow is=\"true\"><mi is=\"true\" mathvariant=\"normal\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></span></span><script type=\"math/mml\"><math><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></script></span> have the largest driving force towards the Fe-rich phase; ion radii and acid/base properties of the different acceptor dopants play a secondary role. The co-segregation of acceptors and <span><span style=\"\"></span><span data-mathml='<math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">&#x2022;</mo><mo is=\"true\">&#x2022;</mo></mrow></msubsup></math>' role=\"presentation\" style=\"font-size: 90%; display: inline-block; position: relative;\" tabindex=\"0\"><svg aria-hidden=\"true\" focusable=\"false\" height=\"2.779ex\" role=\"img\" style=\"vertical-align: -1.043ex;\" viewbox=\"0 -747.2 1558.3 1196.3\" width=\"3.619ex\" xmlns:xlink=\"http://www.w3.org/1999/xlink\"><g fill=\"currentColor\" stroke=\"currentColor\" stroke-width=\"0\" transform=\"matrix(1 0 0 -1 0 0)\"><g is=\"true\"><g is=\"true\"><use xlink:href=\"#MJMAIN-56\"></use></g><g is=\"true\" transform=\"translate(750,306)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g><g is=\"true\" transform=\"translate(353,0)\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-2219\"></use></g></g><g is=\"true\" transform=\"translate(750,-335)\"><g is=\"true\"><use transform=\"scale(0.707)\" xlink:href=\"#MJMAIN-4F\"></use></g></g></g></g></svg><span role=\"presentation\"><math xmlns=\"http://www.w3.org/1998/Math/MathML\"><msubsup is=\"true\"><mi is=\"true\" mathvariant=\"normal\">V</mi><mrow is=\"true\"><mi is=\"true\" mathvariant=\"normal\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></span></span><script type=\"math/mml\"><math><msubsup is=\"true\"><mi mathvariant=\"normal\" is=\"true\">V</mi><mrow is=\"true\"><mi mathvariant=\"normal\" is=\"true\">O</mi></mrow><mrow is=\"true\"><mo is=\"true\">•</mo><mo is=\"true\">•</mo></mrow></msubsup></math></script></span> into the ferrate phase unfortunately decreases the hydration ability of the Ce-rich proton conductor phase. Analogous trends are expected for related proton- and hole-conductor perovskite composites, which partially counteracts the intended mixed conductivity.","PeriodicalId":238,"journal":{"name":"Acta Materialia","volume":"16 1","pages":""},"PeriodicalIF":8.3000,"publicationDate":"2025-01-12","publicationTypes":"Journal Article","fieldsOfStudy":null,"isOpenAccess":false,"openAccessPdf":"","citationCount":"0","resultStr":"{\"title\":\"Electronic structure, phase formation, and defect distribution in the Ba(Ce,Fe,Acc)O3-δ system\",\"authors\":\"R. Merkle, M.F. Hoedl, A. Chesnokov, D. Gryaznov, E. Bucher, E.A. Kotomin, W. Sitte, J. Maier\",\"doi\":\"10.1016/j.actamat.2025.120739\",\"DOIUrl\":null,\"url\":null,\"abstract\":\"Composites of two perovskites are one possibility to combine protonic and p-type electronic conductivity as required for oxygen electrodes in protonic ceramic electrochemical cells. The BaCeO<sub>3</sub>-BaFeO<sub>3</sub> system can be acceptor-doped to increase proton uptake and transport. However, preceding experiments [C. Berger et al., J. Mater. Chem. A 10 (2022) 2474; C. Nader et al., Solid State Ionics 406 (2024) 116474] indicated that the dopants are inhomogeneously distributed between the two phases, which is decisive for hydration ability and proton conductivity of such composites. Here, we use extended density functional theory calculations (DFT+U, Hubbard approach) for a comprehensive characterization of the BaCeO<sub>3</sub>-BaFeO<sub>3</sub> system including acceptors. Supercells of various compositions are calculated to derive chemical reaction energies, for example for the transfer of defects between the phases. Two key aspects related to the hydration ability of such materials are: (i) The development of the electronic structure with increasing Fe content in a (hypothetical) single-phase BaCe<sub>1-x</sub>Fe<sub>x</sub>O<sub>3</sub> perovskite. (ii) The distribution of acceptors (Ga<sup>3+</sup>, Sc<sup>3+</sup>, In<sup>3+</sup>, Y<sup>3+</sup>) and oxygen vacancies (<span><span style=\\\"\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">&#x2022;</mo><mo is=\\\"true\\\">&#x2022;</mo></mrow></msubsup></math>' role=\\\"presentation\\\" style=\\\"font-size: 90%; display: inline-block; position: relative;\\\" tabindex=\\\"0\\\"><svg aria-hidden=\\\"true\\\" focusable=\\\"false\\\" height=\\\"2.779ex\\\" role=\\\"img\\\" style=\\\"vertical-align: -1.043ex;\\\" viewbox=\\\"0 -747.2 1558.3 1196.3\\\" width=\\\"3.619ex\\\" xmlns:xlink=\\\"http://www.w3.org/1999/xlink\\\"><g fill=\\\"currentColor\\\" stroke=\\\"currentColor\\\" stroke-width=\\\"0\\\" transform=\\\"matrix(1 0 0 -1 0 0)\\\"><g is=\\\"true\\\"><g is=\\\"true\\\"><use xlink:href=\\\"#MJMAIN-56\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(750,306)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(353,0)\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g></g><g is=\\\"true\\\" transform=\\\"translate(750,-335)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-4F\\\"></use></g></g></g></g></svg><span role=\\\"presentation\\\"><math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">V</mi><mrow is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></span></span><script type=\\\"math/mml\\\"><math><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></script></span>) between Ce- and Fe-rich phases. The segregation driving forces of acceptor dopant and <span><span style=\\\"\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">&#x2022;</mo><mo is=\\\"true\\\">&#x2022;</mo></mrow></msubsup></math>' role=\\\"presentation\\\" style=\\\"font-size: 90%; display: inline-block; position: relative;\\\" tabindex=\\\"0\\\"><svg aria-hidden=\\\"true\\\" focusable=\\\"false\\\" height=\\\"2.779ex\\\" role=\\\"img\\\" style=\\\"vertical-align: -1.043ex;\\\" viewbox=\\\"0 -747.2 1558.3 1196.3\\\" width=\\\"3.619ex\\\" xmlns:xlink=\\\"http://www.w3.org/1999/xlink\\\"><g fill=\\\"currentColor\\\" stroke=\\\"currentColor\\\" stroke-width=\\\"0\\\" transform=\\\"matrix(1 0 0 -1 0 0)\\\"><g is=\\\"true\\\"><g is=\\\"true\\\"><use xlink:href=\\\"#MJMAIN-56\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(750,306)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(353,0)\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g></g><g is=\\\"true\\\" transform=\\\"translate(750,-335)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-4F\\\"></use></g></g></g></g></svg><span role=\\\"presentation\\\"><math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">V</mi><mrow is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></span></span><script type=\\\"math/mml\\\"><math><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></script></span> are calculated individually. <span><span style=\\\"\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">&#x2022;</mo><mo is=\\\"true\\\">&#x2022;</mo></mrow></msubsup></math>' role=\\\"presentation\\\" style=\\\"font-size: 90%; display: inline-block; position: relative;\\\" tabindex=\\\"0\\\"><svg aria-hidden=\\\"true\\\" focusable=\\\"false\\\" height=\\\"2.779ex\\\" role=\\\"img\\\" style=\\\"vertical-align: -1.043ex;\\\" viewbox=\\\"0 -747.2 1558.3 1196.3\\\" width=\\\"3.619ex\\\" xmlns:xlink=\\\"http://www.w3.org/1999/xlink\\\"><g fill=\\\"currentColor\\\" stroke=\\\"currentColor\\\" stroke-width=\\\"0\\\" transform=\\\"matrix(1 0 0 -1 0 0)\\\"><g is=\\\"true\\\"><g is=\\\"true\\\"><use xlink:href=\\\"#MJMAIN-56\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(750,306)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(353,0)\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g></g><g is=\\\"true\\\" transform=\\\"translate(750,-335)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-4F\\\"></use></g></g></g></g></svg><span role=\\\"presentation\\\"><math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">V</mi><mrow is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></span></span><script type=\\\"math/mml\\\"><math><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></script></span> have the largest driving force towards the Fe-rich phase; ion radii and acid/base properties of the different acceptor dopants play a secondary role. The co-segregation of acceptors and <span><span style=\\\"\\\"></span><span data-mathml='<math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">&#x2022;</mo><mo is=\\\"true\\\">&#x2022;</mo></mrow></msubsup></math>' role=\\\"presentation\\\" style=\\\"font-size: 90%; display: inline-block; position: relative;\\\" tabindex=\\\"0\\\"><svg aria-hidden=\\\"true\\\" focusable=\\\"false\\\" height=\\\"2.779ex\\\" role=\\\"img\\\" style=\\\"vertical-align: -1.043ex;\\\" viewbox=\\\"0 -747.2 1558.3 1196.3\\\" width=\\\"3.619ex\\\" xmlns:xlink=\\\"http://www.w3.org/1999/xlink\\\"><g fill=\\\"currentColor\\\" stroke=\\\"currentColor\\\" stroke-width=\\\"0\\\" transform=\\\"matrix(1 0 0 -1 0 0)\\\"><g is=\\\"true\\\"><g is=\\\"true\\\"><use xlink:href=\\\"#MJMAIN-56\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(750,306)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g><g is=\\\"true\\\" transform=\\\"translate(353,0)\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-2219\\\"></use></g></g><g is=\\\"true\\\" transform=\\\"translate(750,-335)\\\"><g is=\\\"true\\\"><use transform=\\\"scale(0.707)\\\" xlink:href=\\\"#MJMAIN-4F\\\"></use></g></g></g></g></svg><span role=\\\"presentation\\\"><math xmlns=\\\"http://www.w3.org/1998/Math/MathML\\\"><msubsup is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">V</mi><mrow is=\\\"true\\\"><mi is=\\\"true\\\" mathvariant=\\\"normal\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></span></span><script type=\\\"math/mml\\\"><math><msubsup is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">V</mi><mrow is=\\\"true\\\"><mi mathvariant=\\\"normal\\\" is=\\\"true\\\">O</mi></mrow><mrow is=\\\"true\\\"><mo is=\\\"true\\\">•</mo><mo is=\\\"true\\\">•</mo></mrow></msubsup></math></script></span> into the ferrate phase unfortunately decreases the hydration ability of the Ce-rich proton conductor phase. Analogous trends are expected for related proton- and hole-conductor perovskite composites, which partially counteracts the intended mixed conductivity.\",\"PeriodicalId\":238,\"journal\":{\"name\":\"Acta Materialia\",\"volume\":\"16 1\",\"pages\":\"\"},\"PeriodicalIF\":8.3000,\"publicationDate\":\"2025-01-12\",\"publicationTypes\":\"Journal Article\",\"fieldsOfStudy\":null,\"isOpenAccess\":false,\"openAccessPdf\":\"\",\"citationCount\":\"0\",\"resultStr\":null,\"platform\":\"Semanticscholar\",\"paperid\":null,\"PeriodicalName\":\"Acta Materialia\",\"FirstCategoryId\":\"88\",\"ListUrlMain\":\"https://doi.org/10.1016/j.actamat.2025.120739\",\"RegionNum\":1,\"RegionCategory\":\"材料科学\",\"ArticlePicture\":[],\"TitleCN\":null,\"AbstractTextCN\":null,\"PMCID\":null,\"EPubDate\":\"\",\"PubModel\":\"\",\"JCR\":\"Q1\",\"JCRName\":\"MATERIALS SCIENCE, MULTIDISCIPLINARY\",\"Score\":null,\"Total\":0}","platform":"Semanticscholar","paperid":null,"PeriodicalName":"Acta Materialia","FirstCategoryId":"88","ListUrlMain":"https://doi.org/10.1016/j.actamat.2025.120739","RegionNum":1,"RegionCategory":"材料科学","ArticlePicture":[],"TitleCN":null,"AbstractTextCN":null,"PMCID":null,"EPubDate":"","PubModel":"","JCR":"Q1","JCRName":"MATERIALS SCIENCE, MULTIDISCIPLINARY","Score":null,"Total":0}
引用次数: 0
引用
批量引用

 求助内容:
求助内容: 应助结果提醒方式:
应助结果提醒方式:


